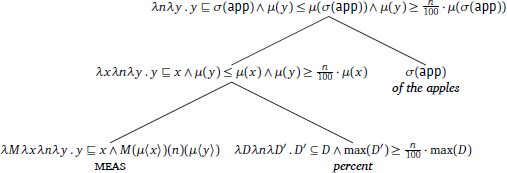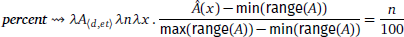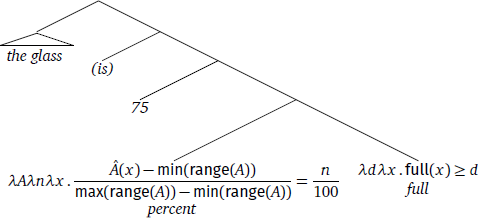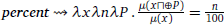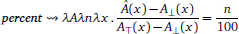1 Introduction

As originally observed by Ahn (2012) for Korean, there are at least two distinguishable uses of so-called proportional measure nouns like percent.1 Sauerland (2014) calls these readings conservative and reversed, respectively (adapted from Sauerland’s (2014) attested examples):

    1. (1)
    1. The most recent class of NASA consists of 50% of the women.            (conservative)
    1. (2)
    1. The most recent class of NASA consists of 50% women.                            (reversed)

Ahn & Sauerland (2015; 2017) show that the alternation is found in German, Korean, French, and English. In the context of the larger cross-linguistic exploration of this special issue, this paper is dedicated to a detailed empirical investigation of the distribution of percent in English using plenty of corpus examples, with an eye toward pushing our theoretical understanding of the phenomenon forward.

The two uses of percent in (1) and (2) both characterize a situation in which there is a part and a whole, where the part makes up some percent of the whole. In (1) (with 50% of the women), the whole is denoted by the women, and there is a part of that whole making up 50% of it that is denoted by the expression 50% of the women. On the other hand, in (2), with 50 women, what comes after percent is a description of the part – there is a larger whole and the part making up 50% of it is characterized as being made up of women. Thus, what immediately follows percent in the ‘reversed’ case is a predicate that characterizes a part of the whole. In the ‘conservative’ case, what immediately follows percent characterizes the whole.

Another place where percent can introduce a predicate that characterizes a part of a larger whole is in predicative sentences with adjectives or with nouns:

    1. (3)
    1. A school system that in 1963 had been 62 percent white became 60 percent black by 1975.                (from COCA)
    1. (4)
    1. The solution is 30% acid.                                                                                                        (adapted from BNCWeb)

In (3), a subpart of the student body making up 62% of it in 1963 is characterized as white, and a subpart making up 60% of it in 1975 is characterized as black. In (4), a subpart of the solution making up 30% of it is characterized as acid. Insofar as what comes after percent characterizes the part, these uses are like ‘reversed’ uses. Indeed, (4) could be rephrased analogously to (2) above:

    1. (5)
    1. The solution consists of 30% acid.

If (2) counts as an example of a reversed use, then so must (5), as it uses the same verb. But because predicative structures are quite different from structures involving transitive verbs, it’s a bit odd to refer to examples like (4) as ‘reversed’ ones.2 In a predicative structure like (4), we would expect 30 acid to have type ⟨e, t⟩, rather than serving as a quantificational noun phrase, as under the Pasternak & Sauerland (2022) analysis of 30% women. The copula does not normally combine with quantifiers (*The class is few students). I will use the term part.introducing to describe both the reversed uses as in (2) and predicative examples like (4) (the idea being, again, that percent precedes, or introduces, a description of the part).

After a tour through some corpus examples of part-introducing uses, I show that existing theories do not immediately capture all of them, and offer a uniform treatment, treating the predicative uses as basic. First, I propose a type-shift that converts a non-gradable predicate to a gradable one that tracks mereological parthood. This makes any non-gradable predicate eligible for use with Pasternak’s (2019) analysis of percent in constructions like 75% full, although it must be modified slightly in order to give the right truth conditions. Second, I sketch a dynamic theory based on Keshet’s (2019) PLural Update Semantics, in which plural discourse referents that summarize the accumulated constraints on a given discourse referent can serve as the ‘whole’ for a percent phrase that both introduces and serves as a predicate. This approach has a number of promising features, including the ability to shed light on certain cumulative-like readings that otherwise elude a compositional treatment.

2 Part-introducing uses

2.1 Predicative constructions

On the view that I will put forward, the most basic configuration in which percent has part-introducing uses is in predicative constructions. By ‘predicative constructions’, I mean ones in which a verb selects an expression denoting a predicate of individuals (type ⟨e, t⟩ or, in the case of gradable predicates, type ⟨d, ⟨e, t⟩⟩). There are a number of predicate-selecting verbs; these include not only the copula but also become, remain, and others. There are two different types of predicative constructions involving percent-modified predicates, which I’ve labeled ‘part-introducing’ and ‘scalar’. These will be exemplified in turn.

2.1.1 Part-introducing predicative uses

‘Part-introducing’ uses involve a non-gradable predicate, and carry the implication that this predicate applies to n% of the subject. These are attested with a range of verbs that accept adjectival complements:3

    1. (6)
    1. a.
    1. … playing the Apollo in New York. The audience was I’d say 90 percent black.
    1.  
    1. b.
    1. Towns such as Albertville … became 34 percent Hispanic.
    1.  
    1. c.
    1. Ole Miss remains 90 percent white.
    1.  
    1. d.
    1. Galileo’s sales have gone 30 percent non-military to 94 percent today.

The examples in (6) have entailments that can be expressed using partitives making reference to subparts of the subject:

    1. (7)
    1. a.
    1. (6a) ⟹ 90% of the people in the audience were black.
    1.  
    1. b.
    1. (6b) ⟹ It became the case that 34% of the people in Albertville were Hispanic.
    1.  
    1. c.
    1. (6c) ⟹ It remained the case that 90% of the people at Ole Miss were white.
    1.  
    1. d.
    1. (6d) ⟹ 94% of the units of Galileo’s sales are now non-military.

In each case, I’ve made artistic decisions about what sorts of things the parts are made up of. I chose people for ‘the audience’, ‘Albertville’, ‘Ole Miss’.I left it open what the units of sales are; they might be transactions, or they might be dollars. Regardless of what the parts are made up of, in every example in (6), the non-gradable predicate following percent applies distributively to each individual part in a collection of subparts of a whole making up the indicated percentage of the whole. Furthermore, the whole is rather evenly divided up into these parts. The whole is denoted by the subject of the verb in these predicative contructions.

In other cases where the verb selects a predicate, the predicate is a noun phrase instead of an adjective phrase, and the noun phrase can be ‘part-introducing’ too. Examples in this category include (4) above as well as the following (again from the COCA corpus):

    1. (8)
    1. After I did Playgirl, my audience became 95 percent white women.
    1. (9)
    1. It must be labeled 100 percent blue agave

As with the cases above involving adjectives, these examples have entailments involving partitives making reference to subparts of the subject:

    1. (10)
    1. My audience became such that 95% of the members of it were white women.
    1. (11)
    1. It must be labelled such that 100% of the parts of it are blue agave

Nominal complements of percent are usually either plural or mass. But it is possible to find singular complements of percent in this category:

    1. (12)
    1. “I’m 85 to 90 percent a different player from the beginning of the season to [now],” Wright said.

This can also be paraphrased with reference to subparts:

    1. (13)
    1. 85 to 90% of the parts of [me] are a different player.

Granted, some of these constructed examples are a bit clunky examples of semanticist-ese, but the intuition seems clear that they are entailed by the corresponding attested sentences. Schematically, predicative part-introducing examples are all cases where (14a) entails (14b).

    1. (14)
    1. a.
    1. SUBJ VERB NUM% PRED
    1.  
    1. b.
    1. SUBJ VERB {such, the case} that NUM% of the [parts of] SUBJ are/were PRED

Here, PRED can be an adjectival or nominal phrase (possibly other categories as well), and what sorts of things the parts are can vary depending on the nature of SUBJ.

2.1.2 Scalar predicative uses

Here are some examples I call ‘scalar’, taken from the COCA corpus:

    1. (15)
    1. a.
    1. the weapon, dating from the 1570s, was packed 70 percent full with gunpowder, sealed with mud and stored up on the Wall
    1.  
    1. b.
    1. Ten minutes of searching your memory to try to pull up what it was you had seen, and after 10 minutes, you said [you were] 40 percent sure. Right?
    1.  
    1. c.
    1. The creators extended the work, while remaining 100 percent faithful to the original, and provided an ending that adds a little something extra…

These cases are akin to cases like 75% full, discussed by Pasternak (2019): The gradable adjective introduces a totally-closed scale, and the value that the subject has on that scale is at the n% mark, with no implications about the status of any of its mereological parts. Unlike the part-introducing cases below, the examples in (15) do not have entailments regarding the subparts of the subject of predication:

    1. (16)
    1. a.
    1. (15a) ⇏ 70 percent of the parts of the weapon were full with gunpowder
    1.  
    1. b.
    1. (15b) ⇏ 40 percent of the parts comprising you were sure
    1.  
    1. c.
    1. (15c) ⇏ 100 percent of the people among the creators were faithful to the original

To say that the weapon is 70% full is not to say that there is a way of dividing the weapon into equal parts such that 70% of those parts have the property of being full. Being 40% sure is not being such that 40% of the parts constituting you are sure; it is having a confidence level corresponding to 40%. Similarly, remaining 100% faithful to the original does not mean being divisible into parts such that each part of you is faithful to the original. If the creators remained 99% faithful to the original, then this does not mean that there was a part of the creators that was not faithful to the original, but rather that there was a part of the work that was not. So the faithfulness scale is not tied to parthood of the creators, the subject of the predicate.

There are several cases for which ‘scalar’ and ‘part-introducing’ analyses are both possible, such as the following:

    1. (17)
    1. And I say we because I feel 100 percent American. I was born and raised here.

This might be said to entail that I feel that 100% of the parts of me are American, but it might not be intended that way, implying merely something like I feel I’m at the 100% mark on the ‘American’ scale. Indeed, one might wonder whether every part-introducing case could be seen as a scalar case, given that it is always possible to create a scale that tracks the part-whole relation, having a maximum endpoint corresponding to the whole. I’ve chosen to label the cases where a mereological part interpretation is plausible as ‘part-introducing’, and cases where it is implausible as ‘scalar’, because the two classes are associated with different entailments. But under the analysis to be proposed, these uses are linked. Below, I will propose to account for the difference by using the theoretical assumption that some adjectives are fundamentally gradable (like full), while others (like Democratic) are fundamentally non-gradable and acquire a gradable interpretation through a type-shifting mechanism that tracks part-hood. In fact, I will propose that the same mechanism is at work in the ‘reversed’ uses.

2.2 Complex attributive modifiers

2.2.1 Attributive part-introducing uses of percent

We turn now to cases involving regular transitive verbs, expressing relations between individuals, instead of selecting a predicate. To set the stage, observe that there is a subtle difference between these two cases:

    1. (18)
    1. Victoria’s Secret… is under pressure to use 50 percent recycled paper in its millions of mailed catalogs and shun paper derived from wilderness regions
    1. (19)
    1. tobacco farmers won a provision in the recent budget bill requiring manufacturers to use 75 percent domestic tobacco

Both contain a string of the form VERB NUM% ADJ NOUN and can be paraphrased using ‘NUM% ADJ’ as a predicate:

    1. (20)
    1. The paper VS uses must be 50% recycled.
    1. (21)
    1. The tobacco manufacturers use must be 75% domestic.

But the similarities end here. It is more natural to paraphrase (18) by replacing 50 percent recycled paper with paper that is 50 percent recycled than it is to paraphrase (19) by replacing 75 percent domestic tobacco with tobacco that is 75% domestic. Another way of looking at it is that 50% recycled is recognizable as a type of paper, but 75% domestic is not recognizable as a type of tobacco. (On the other hand, domestic tobacco is a type of tobacco.) Related: Paper is sold with the label ‘50% recycled’, and tobacco is not sold with the label ‘75% domestic’; it’s sold either as domestic or as imported. Furthermore, the requirement described in (19) is that 75% of the tobacco used by manufacturers is domestic. In (18), in contrast, it sounds as if the requirement is that all of the paper used (in the catalogs) is 50% recycled. For these reasons, I classify (18) as a case where ‘NUM% ADJ’ serves as an attributive modifier of the the noun:

    1. (22)
    1. use [np [ap 50 percent recycled ] paper ]

On the other hand, (19) seems to be a true ‘reversed’ case, the kind that could be analyzed as having focus on domestic.

Among these ‘attributive’ cases, a distinction can be drawn between ‘part-introducing’ and ‘scalar’ uses, just as in the realm of predicative uses of adjectives. Example (18) is a ‘part-introducing’ use; the adjective recycled is not gradable, and there is a way of dividing this paper up into small, evenly-sized parts such that 50% of those parts have the property of being ‘recycled’. Here are some further examples I would place in this category:

    1. (23)
    1. a.
    1. Hey, firefighters can’t afford 100 percent jumbo lump crab meat, OK?
    1.  
    1. b.
    1. Runners need at least three to six one-ounce servings of whole grains per day, and eating 100 percent whole-grain bread (as opposed to just whole-grain bread, which may contain some refined grains and flours) is an easy way to meet this requirement since one slice equals one serving.

Both cases just given involve mass nouns, which do not require determiners. Below are cases where the modified noun is a count noun. In these, the noun phrase has an indefinite determiner a preceding the percent phrase:

    1. (24)
    1. a.
    1. In one study, drinkers and smokers “spontaneously abstained” after eating a 67 percent raw diet.
    1.  
    1. b.
    1. In 1980–81, 5,000 white students in Henry schools created a 75 percent white system.

Here is a case in which the modifier of the count noun is itself a noun:

    1. (25)
    1. But I found a 23 percent hydrochloric acid cleanser.

All of these can be paraphrased using a relative clause: ‘crab meat that is 100% jumbo lump’, ‘bread that is 100% whole-grain’, ‘diet that is 67% raw’, ‘system that is 75% white’, ‘cleanser that is 23% hydrochloric acid’. This shows that in these strings of the form VERB (a) NUM% ADJ NOUN, the string NUM% ADJ functions as an intersective, attributive modifier of NOUN.

The same types of examples can be found in subject position:

    1. (26)
    1. a.
    1. Can a 98% white town attract and keep black, Asian and Hispanic families?
    1.  
    1. b.
    1. After London, the 100% recyclable sculpture made the rounds on a tour of the U.K.
    1.  
    1. c.
    1. Actual rehab of the 95% occupied building took 18 months and $5 million.

These have paraphrases using relative clauses as well: a town that is 98% white; a sculpture that is 100% recyclable; a building that is 95% occupied.

Paraphrases with a relative clause of this kind support the idea that NUM% ADJ serves as an attributive modifier of NOUN, but what shows that these cases are part-introducing attributive modifiers? The parenthetical clarification in example (23b) clearly reveals that a mereological scale is involved in this case. A general way of showing this is to use the same kind of transformations on the sentence that we used in the predicative construction:

    1. (27)
    1. (18) ⟹ VS is under pressure to use paper such that 50% of the parts constituting it are recycled.

Again, it sounds a bit clunky, but the entailment is there. Similar observations hold for the other examples in this section. Thus, in these cases, (28a) can be paraphrased as (28b).

    1. (28)
    1. a.
    1. SUBJ VERB (DET) NUM% ADJ NOUN
    1.  
    1. b.
    1. SUBJ VERB (DET) NOUN such that NUM% of the parts constituting it are ADJ

2.2.2 Attributive uses of percent with scalar complements

In another class of cases involving attributive modifiers of the form ‘NUM% ADJ’, ADJ is a gradable adjective like full. Here are some constructed examples:

    1. (29)
    1. a.
    1. Marie Kondo recommends having 90% full drawers. (drawers that are 90% full)
    1.  
    1. b.
    1. To make this, I create a 50% transparent image in Photoshop. (an image that is 50% transparent)

Like the attributive part-introducing cases, these can be paraphrased with relative clauses, as noted in conjunction with the examples. Moreover, these uses carry analogous entailments to the ones observed for scalar predicative cases:

    1. (30)
    1. a.
    1. (29a) ⟹ MK recommends having drawers that are 90% of the way up on the ‘full’ scale.
    1.  
    1. b.
    1. (29b) ⟹ I create an image that 50% of the way up on the ‘transparent’ scale.

and do not have entailments analogous to the part-introducing cases:

    1. (31)
    1. a.
    1. (29a) ⇏ MK recommends having drawers such that 90% of their parts are full. (drawers that are 90% full)
    1.  
    1. b.
    1. (29b) ⇏ I create an image such that 50% of its parts are transparent.

Here are some attested examples from the COCA corpus in this category:

    1. (32)
    1. With a few tweaks, citrus industry officials say they’ll be able to turn the cameras toward Brazil and get a 95 percent accurate estimate of that country’s orange harvest.
    1. (33)
    1. for three weeks, he spent four to five hours expanding his tough-minded [np [ap 200 percent nationalistic ] theory ]

Example (32) does not imply that the estimate in question can be divided up into parts such that 95% of them are accurate; rather, 95% expresses the degree of accuracy that the estimate enjoys. (33) does not imply that 200% of the (parts of the) theory is (are) nationalistic (if that even made sense). This example seems to involve hyperbole, as nothing can be more than 100% on any scale (although a quantity can be more than 100% of another quantity, as in 200% zoom), but it is clear how to interpret it: The degree of nationalism was way off the charts.

I did not happen to find any clear examples of scalar attributive uses in subject position in the COCA corpus, but they are not difficult to construct:

    1. (34)
    1. A 95 percent accurate estimate of Brazil’s orange harvest can be obtained using this technique.

Thus, it seems that like part-introducing strings of the form NUM% ADJ, scalar ones can function either predicatively or attributively, and when they function attributively, they can modify a noun in any grammatical position.

To summarize: In scalar attributive cases, (28a) can be paraphrased as (35) and cannot be paraphrased as (28b).

    1. (35)
    1. SUBJ VERB (DET) NOUN that is NUM% of the way up on the ADJ scale

2.3 Reversed readings

2.3.1 Uses of percent with reversed readings

We turn now to attested examples of genuine ‘reversed’ readings, like (19), repeated here:

    1. (36)
    1. tobacco farmers won a provision in the recent budget bill requiring manufacturers to use 75 percent domestic tobacco

In these cases, (28a) can be paraphrased as (37a) and/or (37b).

    1. (37)
    1. a.
    1. NUM% of what SUBJ VERB was ADJ NOUN
    1.  
    1. b.
    1. NUM% of the NOUN SUBJ VERB was ADJ

These two paraphrases correspond to the two possible placements of focus under Pasternak & Sauerland’s (2022) theory. The first corresponds to placement of focus on the ‘ADJ NOUN’ combination, and the second corresponds to focus on the ‘ADJ’ combination.

Here are some ambiguous cases:

    1. (38)
    1. The Media Research Center recently documented that Romney got 86 percent negative coverage during his trip to England, Israel and Poland last week.
    2. ‘86% of what Romney got was negative coverage’
    3. or ‘86% of the coverage Romney got was negative’
    1. (39)
    1. he ran 95 percent negative ads, he and his PAC, down in Florida
    2. ‘95% of what he ran was negative ads’
    3. or ‘95% of the ads he ran were negative’
    1. (40)
    1. These locations receive 100 percent fresh air from the building’s bulk air handling system.
    2. ‘100 percent of what these locations receive is fresh air’
    3. or ‘100 percent of the air these locations receive is fresh’
    1. (41)
    1. Google and Yahoo both lost ground, with each company holding 16 percent pay-per-click shares for the first six months of 2006.
    2. ‘16 percent of what each company held was pay-per-click shares’
    3. or ‘16 percent of the shares each company held were pay-per-click’

In these cases, the second paraphrase is more precise, but the first paraphrase is also possible, as long as there is some room for contextual narrowing on the subcategorization requirements of the verb. Given an interpretation of the verb get that is restricted in context to the kinds of things that the media gives—namely coverage—then in context, ‘what Romney got’ is the same as ‘the coverage Romney got’. So these two readings collapse. Analogous observations hold for the other examples in this set.

There are also unambiguous cases, where the ADJ-focus paraphrase is clearly unavailable:

    1. (42)
    1. The only difference was that the first group’s food contained 1 percent linoleic acid (consistent with the U.S. diet circa 1900) while the second group…
    2. ‘1% of what the first group’s food contained was linoleic acid’
    3. (not: ‘1% of the acid the first group’s food contained was linoleic’)
    1. (43)
    1. Just as important, the location offered access to raw materials like bog iron ore, which workers collected from the bottom of bogs, swamps, ponds, and riverbeds, yielding a mixture of materials that contained 30–50 percent usable iron.
    2. ‘30–50% of what the materials contained was usable iron’
    3. (not: ‘30–50% of the iron the materials contained was usable’)
    1. (44)
    1. The cosmos holds 73 percent dark energy, 23 percent dark matter, and a measly 4 percent ordinary matter
    2. ‘73% of what the cosmos holds is dark energy’
    3. (not: ‘73% of the energy the cosmos holds is dark’)
    1. (45)
    1. … campaign directed at Coca-Cola, which made—and failed to keep—a 1990 promise to use 25 percent post-consumer waste in its plastic beverage bottles
    2. ‘promise that 25% of what they use is post-consumer waste’
    3. (not: ‘promise that 25% of the waste they use is post-consumer)

It may be worth noting that in many of these particular examples, ‘NUM % of what x VERB’ is equivalent to ‘NUM % of x’: ‘1% of what the first group’s food contained’ is equivalent to ‘1% of the first group’s food’. Hold is similar to contain: ‘73% of what the cosmos holds’ is equivalent to ‘73% of the cosmos’. This equivalence does not always hold, though: ‘25% of what Coca-Cola uses’ is not the same as ‘25% of Coca-Cola’.

The following case involves quantification over the subject position, and each witness for the quantifier corresponds to its own reversed reading.

    1. (46)
    1. Until last year, Beijing required that wind turbines sold in China contain 70 percent Chinese-made parts.
    2. ‘for all wind turbines sold in China x, 70% of what x contains is Chinese-made parts’
    3. or ‘… 70% of the parts x contains are Chinese-made’.

These paraphrases do entail paraphrases of the form ‘NUM% of what SUBJ VERB is ADJ NOUN’ and ‘NUM% of the NOUN SUBJ VERB is ADJ’, respectively (‘… 70% of what wind turbines sold in China contain is Chinese-made parts’; ‘… 70% of the parts wind turbines sold in China contain are Chinese-made’). But they say something more specific too, imposing the same condition on all wind turbines. These observations are entirely expected in light of the fact that quantifiers are scopally mobile, but this case does help to illustrate that the entailment patterns depend on whether SUBJ is quantificational.

Now, in some cases, a reversed interpretation is possible, but so is an attributive interpretation, where ‘NUM% ADJ’ serves as a modifier of NOUN. With attributive uses, recall that the sentence can be restated equivalently as ‘SUBJ VERB NOUN that was NUM% ADJ’. For many cases, both of those paraphrases sound about right, so it’s difficult to make a firm decision about which analysis among those is correct. For example, consider the following:

    1. (47)
    1. Up until now, blame for this kind of corrosion has rested squarely on product imported from China, but the Brinkews say they ordered 100 percent American dry wall.

Did they order dry wall that is 100% American or was 100% of what they ordered American dry wall? Or was 100% of the dry wall they ordered American? All of the above seem to fit reasonably well. This case is thus ambiguous between ‘reversed’ and ‘attributive’ (and between two ‘reversed’ interpretations, corresponding to focus on either ‘ADJ’ or ‘ADJ NOUN’).

2.3.2 Distribution of verbs

Even taking the ambiguous cases into account, (potential cases of) reversed readings are restricted to quite a limited set of verbs. Table 1 shows a list of the verb lemmas that figured in examples categorized as (possibly) ‘reversed’, along with their frequency among the search results and example predicates. The frequency information gives a sense of which verbs are most prototypical for a reversed use. There is a striking similarity among them: In almost all cases, the verb can be replaced by ‘incorporate’ without changing the meaning terribly much.

Table 1

Verbs occurring in reversed constructions involving adjectives in the COCA corpus

verb.lemma frequency example.predicates
contain 23 red clover, organic compound, lean protein
use 19 blue agave, post-consumer waste, actual calfskiin
include 6 American content, Pell-eligible students,
purchase 3 renewable power, renewable energy,
hold 2 dark energy, pay-per-click shares
provide 2 renewable power, renewable energy
show 2 affirmative answers
add 1 green light [to plants]
be fed 1 organic feed
buy 1 clean energy
get 1 negative coverage
go 1 clean energy
harness 1 new oak [in wine production]
incorporate 1 recycled plastic
mix 1 ground-up, dried manure
order 1 American dry wall
receive 1 fresh air
run 1 negative ads
see 1 pure 10s [of women; gross!]
sell 1 Fair Trade coffee
substitute 1 compostible cups

Absence of corpus examples of a particular kind does not prove ungrammaticality, but observe the contrast among the following constructed examples:

    1. (48)
    1. a.
    1.    The committee hired 75% women.
    1.  
    1. b.
    1. ??The committee disliked 75% women.

To my ears, (48b) sounds terrible. While I cannot offer an explanation for this contrast, the acceptability of such sentences does seem to have something to do with an implication of coming into existence (within a given situation); compare:

    1. (49)
    1. a.
    1.    The committee hired some women, so there were more women.
    1.  
    1. b.
    1. #The committee disliked some women, so there were more women.

This is one of the diagnostics that Coppock & Beaver (2015) use for ‘entity-introducing verbs’. Another is the following:

    1. (50)
    1. a.
    1. There are seven women. If the committee hires a woman, that will make eight.
    1.  
    1. b.
    1. There are seven women. #If the committee dislikes a woman, that will make eight.

On the other hand, presentational verbs are not good with percent complements:4

    1. (51)
    1. #There appeared 75% women.

Yet they are arguably ‘entity-introducing’; although the following sentence is not perfectly natural, it does seem to have an entity-introducing implication.

    1. (52)
    1. There are at least seven women. ?If there appears a woman, that will make eight.

So, being ‘entity-introducing’ may be a necessary, but not sufficient condition.

2.4 Subject uses

Before moving on, let us briefly address reversed uses in subject position. Attested uses of percent that could be categorized as ‘reversed’ are not in abundant supply, but here is a sentence that could be understood that way, offered by a reviewer:

    1. (53)
    1. Approximately 10% water will remain.

This could certainly be read as a part-introducing use, insofar as what comes after percent is a predicate that characterizes the 10% part. It’s clearly not predicative, since 10% water is not functioning as the complement of a predicate-selecting verb. It’s also clearly not attributive, because 10% does not combine with an adjectival or nominal modifier to create a complex attributive modifier of a noun. The example can be paraphrased analogously to reversed uses:

    1. (54)
    1. Approximately 10% of what will remain is water.

So this looks like a ‘reversed’ use in subject position.

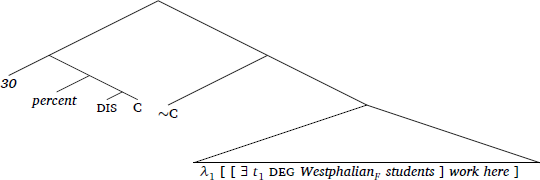
Below, we will review Pasternak & Sauerland’s (2022) analysis of the sentence 30% Westphalian students work here—another example with a reversed reading in subject position. The original example is in German, and it does not feel perfectly natural in English, but I believe it is grammatical and has the relevant reading.

Subject uses can also be obtained by passivizing object uses:

    1. (55)
    1. 25 percent post-consumer waste was supposed to be used in Coca-Cola’s plastic beverage bottles.
    1. (56)
    1. ?75% women were hired by the committee.

The slight degredation of (56) relative to (55) may be due to a subject-verb agreement problem; 75% women does not work well as a plural. (It works even less well as a singular; replacing were with was makes it clearly ungrammatical for me.) But the following sentence strikes me as dramatically worse than (56):

    1. (57)
    1. *?75% women were disliked by the committee.

Thus it seems that the same restrictions on the set of verbs that we saw above applies to subject uses with passive verbs.

Overall, it does seem that reversed uses in subject position are a bit degraded and a bit more difficult to find. This may be due to a requirement for focus on the following predicate (which is predicted under some analyses; see below). As is well-known, English subjects tend to be topics, rather than foci (Erteschik-Shir 2007; Foley 2007; Lambrecht 1994).

2.5 Cumulative readings

The final part-introducing use I will discuss involves something like a cumulative reading (i.a. (Scha 1981; Krifka 1986; Champollion 2009: i.a.):

    1. (58)
    1. In one approach, they mix 10 percent ground-up, dried manure with 90 percent coal (by weight) and then…

(58) doesn’t mean ‘10% of what they mixed with 90% coal was ground-up, dried manure’, so it doesn’t pattern exactly like the reversed examples we’ve considered. I therefore place it in a separate category. It also doesn’t mean ‘They mixed manure that was 10% ground-up and dried with 90% coal’, so an attributive reading seems out of the question for this example. It could be paraphrased as ‘10% of what they mixed in was ground-up, dried manure and 90% of what they mixed in was coal’. This paraphrase does not fit in with any of the categories outlined so far. The example is, however, a part-introducing case, insofar as what immediately follows percent is a characterization of the part.

What makes (58) like a cumulative reading is that the quantificational elements do not take scope independently of each other. A classic example of a cumulative reading is in the sentence 600 Dutch firms own 5000 American computers (Scha 1981), where 600 Dutch firms and 5000 American computers are involved, but for any given firm, it’s not guaranteed that it owns all 5000 computers, nor is it guaranteed that any of the 500 computers are owned by any of the firms. So neither of the quantificational expressions takes scope over the other. As Champollion (2009: 216) puts it, citing Szabolcsi (1997), “[c]umulative readings express information about the cardinalities of the minimal witness sets associated with the quantifiers involved.” In (58), information is expressed about the proportions of the relevant whole made up by the minimal witness sets associated with the quantifiers involved: They mixed x with y, and x makes up 10% of some whole and y makes up 90% of that whole. We’ll see a more detailed rendition of this analysis in section 6.

Here are some additional examples in this category from the COCA corpus, all involving coordination:

    1. (59)
    1. a.
    1. it is composed of 55 percent fructose and 45 percent glucose
    1.  
    1. b.
    1. The 65-gram, 240-calorie breakfast contains 12 percent sodium and 11 percent fat.
    1. (60)
    1. a.
    1. E85 is a mixture of 85 percent ethanol and 15 percent gasoline.
    1.  
    1. b.
    1. Many contractors want a combination of 25 percent compost and 75 percent topsoil for flower beds.

The following cases, using with, are a bit more involved:

    1. (61)
    1. Much less biocide is needed per gallon of paint - typically 25 percent along with 5 percent zinc omadine.
    1. (62)
    1. biodiesel runs fine in unmodified diesel engines at up to a 20 percent blend with 80 percent petroleum diesel, a combination known as B20.

In considering these examples, a question that arises is whether and to what extent conjunction (or comitative semantics) plays an important role in cases like (58). In that example, it seems that the two percent cases are not actually syntactically coordinated:

    1. (63)
    1.   Q: #What did they mix?
    2.   A: #10 percent manure with 90 percent coal.
    1. (64)
    1. #What they mixed was 10 percent manure with 90 percent coal.

Rather, the with-phrase appears to introduce its own syntactic argument in this case. So while these cumulative-like readings often involve coordination, they need not do so.

2.6 Summary

To summarize, we have identified several categories of predicate-selecting percent, each licensing their own paraphrase patterns. A string of the form:

    1. (65)
    1. SUBJ VERB NUM% ADJ (DET) (NOUN)

can be paraphrased in several ways. When VERB is predicative, and there is no determiner DET, there are two possibilities, ‘scalar’ and ‘part-introducing’. In the ‘part-introducing’ case, a paraphrase of the form

    1. (66)
    1. SUBJ VERB {such, the case} that NUM% of the parts of SUBJ are ADJ (NOUN)

is possible. In the ‘scalar’ case, that type of paraphrase is not possible, but one of the following form is:

    1. (67)
    1. SUBJ VERB NUM% of the way up on the ADJ scale

When VERB is an ordinary transitive verb, and a NOUN is present, one possibility is that NUM% ADJ acts an an attributive modifier of NOUN. In that case, a paraphrase with a relative clause is possible:

    1. (68)
    1. SUBJ VERB (DET) NOUN that is NUM% ADJ

Here again, there are two possibilities, scalar and part-introducing. In the part-introducing case, a paraphrase of the following form is possible:

    1. (69)
    1. SUBJ VERB NOUN such that NUM% of the parts of it are ADJ

In the scalar case, a paraphrase of that form is not possible, although one of the following form is:

    1. (70)
    1. SUBJ VERB NOUN that is NUM% of the way up on the ADJ scale

There are also reversed readings, when there is no determiner DET and a NOUN is present. Here again there are two possibilities, but in this case it depends on the placement of focus. When focus is on ADJ, the following paraphrase is possible:

    1. (71)
    1. NUM% of the NOUN SUBJ VERB is ADJ

When focus is on the ‘ADJ NOUN’ combination, it can be paraphrased:

    1. (72)
    1. NUM% of what SUBJ VERB is ADJ NOUN

There are also cumulative-readings, which don’t quite fit into this schema. In the next section, we will apply existing analyses to these cases and see how far we can get.

3 Sauerland & Pasternak

3.1 Conservative uses

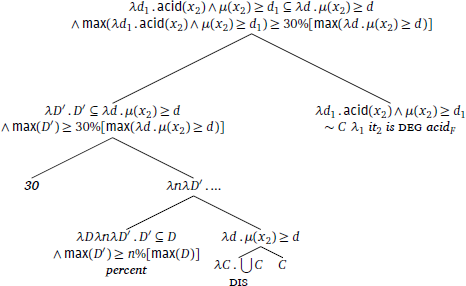
At the time of writing, the most recent analysis that has been made publicly available (Pasternak & Sauerland 2022: available on LingBuzz) treats percent as in (74). This analysis is analogous to their treatment of absolute measure nouns like kilo, which they treat as in (73). Both percent and kilo combine with two degree predicates D and D′ (type ⟨d, t⟩) in addition to a number n (which enters into the composition between the two degree predicates).

    1. (73)
    1. kilo ⟿ λDλnλD′. D′ ⊆ D ∧ max(D′) ≥ n · kg
    1. (74)
    1. percent ⟿ λDλnλD′. D′ ⊆ D ∧ max(D′) ≥

Note that in (73), I have written n·kg to represent the quantity of n kilograms using the dot (·) for multiplication, assuming that degrees and numbers can be multiplied as described by Coppock (2021); I assume kg denotes a particular degree, the one corresponding to one kilogram.

In partitive, ‘conservative’ uses, these measure nouns will be selected by a function they call meas (a bit like Solt’s (2009) meas, but compositionally different), defined as follows.

    1. (75)
    1. meas ⟿ λMλxλnλy . y ⊑ x ∧ M(μ⟨x⟩)(n)(μ⟨y⟩)
    2. where μ⟨α⟩ is defined as λd . d ≤ μ(α)

Here, μ is to be read as a free variable, set by context to an appropriate measure function.5

In a partitive example like 30 kilos of the apples, meas combines first with kilos, as in the following derivation (in which some simplifications are used, including rewriting max(μ⟨x⟩) as μ(x) and restating a subset relation between two degree predicates as a less-than-or-equal relation among their maxima):

    1. (76)

The same happens with meas and percent, giving the following derivation.

    1. (77)

Hence n percent of the apples ends up denoting a property that holds of a subpart of the apples making up n percent of it. If this property combines with a silent existential quantifier, this analysis will yield conservative truth conditions for a sentence like 5 of the apples are ripe, in the sense that this sentence will be equivalent to 5% of the apples are apples that are ripe. With the existential quantifier, the meaning can be paraphrased ‘there is an x such that x makes up 5% of the apples and x is ripe’, which clearly entails that ‘there is an x such that x makes up 5% of the apples and x is ripe apples’.

3.2 Reversed readings

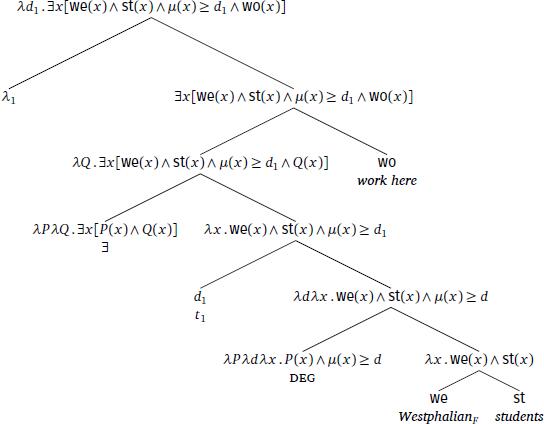
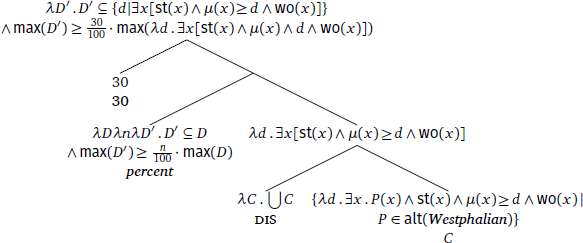
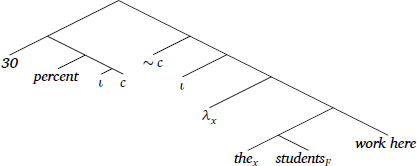
The ‘reversed’ reading is derived from the following structure:

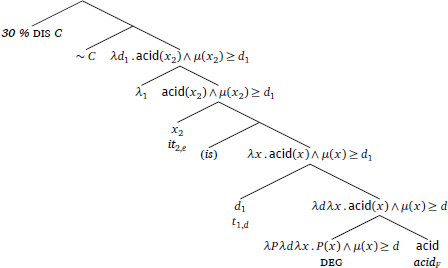
    1. (78)

where dis is short for ‘disjunction’ and acts semantically as a grand union operator that applies to a set. As shown in the following tree, deg converts a non-gradable predicate to a gradable one by introducing a contextually-determined μ function. The meaning that ∼C attaches to is derived as follows:

    1. (79)

Hence the value of C will be:6

    1. (80)
    1. {λd . ∃x .P(x) ∧ st(x) ∧ μ(x) ≥ d ∧ wo(x) | P ∈ alt(Westphalian)}

Assuming, following Pasternak & Sauerland (2022), that the trivial property λx . ⊤ is among the alternatives to Westphalian, the 30 percent dis C component will have semantics derived as follows:

    1. (81)

Simplifying a bit, putting these two subtrees together will produce the following:

    1. (82)
    1. max(λd1 .∃x[we(x) ∧ st(x) ∧ μ(x) ≥ d1 ∧ wo(x)])
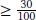    2. · [max(λd . ∃x[st(x) ∧ μ(x) ≥ d ∧ wo(x)])]

Here is another way of saying the same thing:

    1. (83)
    1. |we ∩ st ∩ wo| 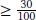· |st ∩ wo|
    2. ‘(At least) 30% of the students who work here are Westphalian’

So this analysis derives truth conditions for a reversed reading in a focus-sensitive manner, using the same lexical entry that is used to derive conservative truth conditions (given the assumption that the trivial property is always among the focus alternatives, and a silent disjunction operator). Notice that the truth conditions for the reversed case are not conservative, in the sense that 30 Westphalian students work here does not entail 30 Westphalian students are Westphalian students who work here.

3.3 Predicative uses

Now let us consider predicative uses like the following:

    1. (84)
    1. The solution is 30% acid.               (adapted from BNCWeb)

These are like reversed cases in that what follows percent is a predicate that holds of a subpart of a whole. Indeed, (84) could be rephrased analogously to (2) above:

    1. (85)
    1. The solution consists of 30% acid.

But I assume that the copula takes a predicative complement, while consists of does not.

If we assume that 30% acid is type ⟨e, t⟩, and that 30% is raised and interpreted as under Pasternak & Sauerland’s (2022) analysis of reversed structures, then we derive the following structure:

    1. (86)

Expanding the 30% dis C part gives:

    1. (87)

In words, the truth conditions derived can be expressed as follows: The degree to which x2 is acid and big (along dimension μ) is greater than or equal to 30% of the degree to which x2 is big (along dimension μ). Since ‘acid’ is a unary predicate under this treatment, a given object will be in its extension or not; there is no middle ground. If x2 is not acid, then the first degree predicate will yield an undefined value, because the maximum of the empty set is undefined. So, assuming that something that is 30% acid is not acid, the sentence is predicted to have a truth value of ‘undefined’ when it is in fact true.

4 Pasternak on 75% full

Is there a precedent in the literature that we could look to in order to come to grips with these simple predicative cases like The solution is 30% acid? Pasternak (2019) provides a theory of some predicative cases involving adjectives like the following:

    1. (88)
    1. The glass is 75% full.                                             (Kennedy & McNally 2005; Pasternak 2019)

These are the types of uses labeled ‘scalar’ above. As Pasternak notes, this construction requires a gradable predicate that is associated with a totally closed scale, i.e., one that has both a minimum and a maximum; cf. #70% tall.

Pasternak defines percent as follows:7

    1. (89)

where:

  • A is a gradable predicate associating individuals with degrees

  • range(A) picks out the set of degrees that A could sensibly associate an individual with—and is defined as λd .∃x . defined(A(d)(x)), following Pasternak

  • defined(ϕ) is true if and only if ϕ is true or false

  • max(D) is equivalent to ιd . ∀ d′[D(d′) → d′ ≥ d]

  • min(D) is equivalent to ιd . ∀ d′[D(d′) → d′ ≤ d]

This lexical entry yields a straightforward analysis of predicative cases like (88):

    1. (90)

The fraction appearing to the left of the equals sign contributed by the lexical entry for percent will have in the numerator the degree to which the glass is full, assuming the min term is the zero element for the ‘fullness’ dimension. (Here I am embellishing on Pasternak’s explanation using the system described by Coppock (2021), following Raposo (2018; 2019), where there are many zeroes, one for each dimension.) The denominator will be the degree corresponding to the size of the full range of possible fullnesses, which will be equal to the maximum possible degree of fullness (assuming again that the minimum is the relevant zero element). Whether or not fullness-degrees are themselves proportions, and in whatever units fullness is measured, this fraction can be represented as a number, since the quotient of two quantities associated with the same dimension (e.g. fullness) is a so-called ‘dimensionless quantity’, representable by a number without any accompanying unit (JCGM 2012; Coppock 2021). (Here again I am embellishing on Pasternak’s explanation.) The truth conditions expressed by that sentence is that the ratio of these two quantities is equal to the number .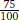

As Pasternak shows, this lexical entry can elegantly be extended to handle conservative cases. Incorporating ideas from Wellwood (2015), Pasternak proposes the following lexical entry for silent much:

    1. (91)
    1. much ⟿ λyλdλx . ∂ (μ(y) ≥ d) ∧ x ⊑ y ∧ μ(x) ≥ d
    2. where ∂(ϕ) is true if ϕ is true, and undefined otherwise.

I have written the presupposition that μ(y) ≥ d using the ‘partial operator’ ∂(Beaver & Krahmer 2001). This presupposition is contributed by Pasternak, and it plays a crucial role in the truth conditions, because it controls the range of the derived gradable predicate.

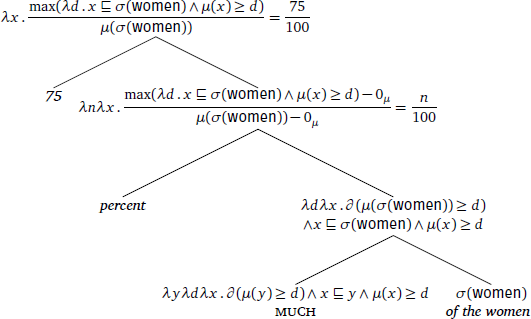
Let us see how it works with a ‘conservative’ example like (48a) (The committee hired 75% of the women). This silent much combines with of the women to produce a gradable predicate that percent then combines with:

    1. (92)

The variable A in the lexical entry for percent is replaced by the following gradable predicate:

  •       λdλx . ∂(μ(σ(women)) ≥ d) ∧ x ⊑ σ(women) ∧ μ(x) ≥ d

Intuitively, this measures the size of a subpart of the women. The greatest degree for which A is defined for anything can be expressed as μ(σ(women)), because of the presupposition requiring that d to be smaller than or equal to the measure of the women μ(σ(women)). The smallest degree is 0μ, “the 0-degree of μ” (p. 78). (In Coppock’s (2021) terms, this would be the additive identity element for the dimension along which μ measures things.) Referring to this element ensures that the scale is lower-bounded.

Pasternak is not 100% explicit in the text about why zero is the lowest degree in the range, but this result could be assumed to follow in part from the fact that d is comparable to μ(x) using ≥, which would mean that it is a degree of the same dimension as μ(x). (I am assuming that every degree/quantity has a corresponding dimension; cf. Raposo’s (2019) dim mapping.) The comparability of those degrees, signalling a common dimension, along with an assumption that there are no degrees below zero along that dimension, could derive the result 0μ is the lowest d for which A(x)(d) is defined for this A and some x.

If we add a silent existential quantifier to 75% of the women, then for (48a), we derive the proposition that there is a plural individual making up 75% of the women that the company hired. Strictly speaking, this does not rule out the possibility that the company hired more than 75% of the women, but that upper-bounding inference may well be pragmatic. Success!

Pasternak (2019) claims that “this analysis can be extended equally well to Ahn & Sauerland’s (2017) treatment of [reverse cases]”. Under Ahn & Sauerland’s (2017) treatment, percent takes an individual as its first argument, representing the ‘whole’. In reversed cases, this argument is saturated by a silent definite description whose descriptive content is (a flattened version of) the focus semantic value of the sentence, abstracting over the position of the percent nominal using ‘modified trace conversion’, which inserts an indexed definite determiner thex in the would-be trace position. Thus (93a) has the Logical Form (LF) in (93b).

    1. (93)
    1. a.
    1. 30% StudentenF
    2. 30% students
    1. arbeiten
    2. work
    1. hier 30% students work here’
    2. here.’
    1.  
    1. b.

Ahn & Sauerland use the following lexical entry for percent:

    1. (94)

One way to merge their treatment of reverse cases with Pasternak’s assumptions might be to combine Pasternak’s lexical entry for percent with Ahn & Sauerland’s (2017) syntactic assumptions, with the exception that a silent much is inserted above ιc so as to produce an argument of type ⟨d, et⟩ that percent could combine with. I believe this would enable a compositional treatment of relative readings that produces the right truth conditions.

This treatment does not immediately account for cases like The solution is 30% acid, though, in part because acid is not a gradable adjective, so it is the wrong type to combine. On the other hand, if acid could be coerced into a gradable predicate, then perhaps a treatment of such cases could be obtained. The question is what sort of coercion operation would yield the right truth conditions.

5 From non-gradable to gradable

5.1 Some non-solutions

Not every way of converting a non-gradable predicate like acid into a gradable one delivers the right truth conditions. Pasternak’s (2019) much is a device we have already used for converting things into gradable predicates, but it expects an argument of type e. Suppose that in order to satisfy that type requirement, we coerced acid into an individual by making it denote the sum of all (contextually-relevant) acid in order to satisfy the type requirements of much. Then we would derive a meaning for The solution is 30% acid that could be rendered back into English as The solution is 30% of the acid. This is not a faithful interpretation.

Alternatively, we might try to convert acid into a gradable property using m.op, from Rett (2018: 105), which shifts an ⟨e,t⟩ meaning to a ⟨d,⟨e,t⟩⟩ meaning:

    1. (95)
    1. m-op ⟿ λPλdλx . P(x) ∧ μ(x) ≥ d
    1. (96)
    1. m-op acid ⟿ λdλx . acid(x) ∧ μ(x) ≥ d

As a minor variant, we could use Solt’s (2009) meas, defined as λxλd . μ(x) ≥ d, and combine it with acid as she proposes using ‘Variable Identification’, which would give the same thing. Whether we derive the meaning in (96) using m.op or meas, the truth conditions for The solution is 30% acid would then be ‘The solution is acid and its measure is 30%.’ This is not correct either.

5.2 A solution

My proposal for how to link 75% full and 30% acid involves converting a non-gradable predicate into a gradable one tracking parthood of whatever it applies to. For the purposes of discussion, let us imagine that there is a silent lexical item called part that denotes the operation in question:

    1. (97)
    1. part ⟿ λPλdλx . ∂(μ(x) ≥ d) ∧ ∃y[ y ⊑ x ∧ μ(y)=d ∧ P(y)]                              ≡part

Combined with a non-gradable predicate like acid, part will yield a gradable predicate that holds of an object to a greater degree the more parts of it satisfy the input predicate. That gradable predicate can then serve as an argument to percent. Our part is similar to much, but it combines first with a predicate like acid first rather than the ‘whole’, and the ‘part’ element (y) is existentially bound; the gradable predicate that it produces after applying to its first argument applies to a whole rather than a part. The partial operator ∂ is used introduce a presupposition inspired by the one in Pasternak’s much. It plays a similar role here: constraining the set of degrees so that they are upper-bounded by the μ-measure of the whole.

To complete the analysis, it will be necessary to modify Pasternak’s percent slightly.8 Applying part to acid yields the following gradable predicate:

    1. (98)
    1. part acid ⟿ λdλx . ∂(μ(x) ≥ d) ∧ ∃y[y ⊑ x ∧ μ(y)=d ∧ acid(y)]

Let us abbreviate this predicate as A. In Pasternak’s lexical entry for percent, the denominator is

  •       max(range(A)) – min(range(A)).

The max expression picks out the greatest element in the range of A. The range of A consists only of values for d such that there is a value for x such that μ(x) ≥ d, since that is a definedness condition, marked by ∂. There are no other definedness conditions, so the range consists of all such values. Suppose μ is understood as weight; then the maximum element of the range is the weight of the heaviest object(s) in the domain. The domain might not contain a heaviest object, in which case the expression is undefined. But even if it is defined, it does not provide the quantity we are looking for, because the denominator should not be the weight of the heaviest object; it should be the weight of the ‘whole’ relative to which we are comparing the weight of the ‘part’.

With the following definition of percent, the ratio in question is between the μ-measure of the acid-part of the whole and the μ-measure of the whole:

    1. (99)

where:

  • A⊥(x) abbreviates min(λd . defined(A)(d)(x)), the least value for d such that A(d)(x) is defined

  • A⊤(x) abbreviates max(λd . defined(A)(d)(x)), the greatest value for d such that A(d)(x) is defined

When percent combines with part acid, the greatest value for d such that A(d)(x) is defined is the greatest value for d such that μ(x) ≥ d is true. Hence, since x stands for the ‘whole’ of which the acid is the ‘part’, the denominator now correctly picks out the relevant monotonic measure of the ‘whole’. Applying the part shift to acid produces the following reasonable-seeming meaning for 30 percent acid:

    1. (100)

Two key moments in this derivation are the simplification of A⊤(x) to μ(x) and the simplification of A⊥(x) to 0μ. In this case A is:

  •       λdλx . ∂(μ(x) ≥ d) ∧ ∃y[ y ⊑ x ∧ μ(y)=d ∧ acid(y)]

The maximum will be μ(x) due to the presupposition, and the minimum will be 0μ assuming that is the smallest degree on the μ dimension, and that A maps the value of x to either true or false at the smallest degree on that dimension. Another important moment is the simplification of

  •       max(λd .∂(μ(x) ≥ d) ∧ ∃y[ y ⊑ x ∧ μ(y)=d ∧ acid(y)])

to μ(σ(λy . y ⊑ x ∧ acid(y))). This is licensed because the greatest degree to which an acid subpart of something measures that degree (on some dimension) is the measure of the mereological sum of the subparts that are acid (on the same dimension). Success!

With this change to Pasternak’s percent, we have not lost an account of 75% full. Recall that for a given predicate A, range(A) is not the set of degrees that A actually does associate an individual with; it the set of sensible degree to associate anything with. So it is reasonable to imagine that any object in the domain will be associated with the same definedness range. Hence, we are free to construct the range of A based on any arbitrary member of the domain, rather than existentially quantifying over it, in order to produce the relevant range for cases like 75% full.

That said, some constraints must be put on this part operation. As a reviewer points out, the distribution of the gradable version of acid under consideration here is limited. It does not combine directly with measure phrases, for example:

    1. (101)
    1. ?The solution is 5mg acid.
    2. (Intended: The acid part of the solution is 5mg.)

Furthermore, as the same reviewer points out, The soup is 30% oil does not mean that the temperature of the oil part of the soup is 30% of the temperature of the soup. In other words, this construction only permits measures that grow monotonically with mereological expansion of an object or substance, going from a part to a whole; things like volume and weight. This observation is reminiscent of the constraints on the monotonicity head mon head posited by Schwarzschild (2006). Perhaps mon is involved here. The reviewer observes that part can be derived from more basic parts including mon and a silent partly:

    1. (102)
    1. mon ⟿ λPλdλx[ P(x) ∧ μ(x) = d]                                                                           ≡mon
    2. where μ is a measure that grows monotonically with mereological expansion
    1. (103)
    1. partly ⟿ λFetλx∃y[ y ⊑ x ∧ F(y)]                                                                         ≡partly

A Geach rule geachd, defined such that geachd (F) = λV⟨d,α⟩λd . F(V(d)), where F is type ⟨α,β⟩, can be applied to partly to yield a function that composes with mon to produce part:

    1. (104)
    1. geachd (partly) ° mon
    2. ≡ λP[geachd(partly)](mon(P))
    3. ≡ λP[geachd(partly)](λdλx[P(x) ∧ μ(x) = d])
    4. ≡ λPλd . partly(λdλx[P(x) ∧ μ(x) = d])
    5. ≡ λPλλx∃y[ y ⊑ x ∧ P(y) ∧ μ(y) = d]
    6. ≡ part

If it is though these more basic operations that the part-tracking gradable predicate is produced, then it should only manifest itself in the syntactic environment of the mon head, and the range of μ-measures that a context might supply should be constrained to those allowed by the mon head (e.g., the montonic ones, if monotonicity is indeed the key property).

This analysis also predicts a mereological/part-introducing reading for The pie is 40% hot, where 40% of the pie is hot. Since the hot scale is not totally closed, we do not get a scalar reading for this example, as Pasternak’s entry was carefully designed to ensure that the only predicates that could appear as the complement to percent are totally closed.

We have just seen that this analysis works well for the predicative cases. The same kind of treatment would also straightforwardly produce the right kind of truth conditions for part-introducing attributive cases like 50% recycled paper; the modifier 50% recycled would translate as an expression of type ⟨e,t⟩ in an entirely analogous way to 30% acid, and this could be combined with paper using Predicate Modification.

Our analysis also predicts that expressions of the form n% P, for some predicate P, should be capable of occurring in a pseudopartitive construction or as the complement of a determiner, since such expressions denote predicates. This prediction is borne out (examples from Google, and confirmed as grammatical by the author’s native speaker intuitions).

    1. (105)
    1. Can I eat a lot of 100 percent dark chocolate without added sugar and still lose weight?
    1. (106)
    1. If much material remains on the filter, an ounce of 50 percent alcohol may be used to dissolve it.
    1. (107)
    1. How much 50 percent wettable powder formulation should be added per tankful of water?
    1. (108)
    1. While most 100 percent fruit juice is 100 percent sugar, you can try tomato juice or a vegetable juice alternative.

Replacing most with all in (108) yields a grammatical example as well. Phrases of the form n% P generally behave predicates, as the analysis predicts.

Pasternak’s (2019) much can be adopted in order to gain a satisfactory compositional account of the ‘conservative’ uses. This option is available under the present proposal as well.

Could we work backwards from this analysis to gain a general account of part-introducing uses, including the reversed cases as in (109)?

    1. (109)
    1. The committee hired 75% women.

Suppose that 75% women, which denotes a predicate on this analysis, could undergo a kind of Quantifier Raising (QR), and that a sum operation took place over the resulting lambda abstract:

    1. (110)

Then the predicate denoted by 75% women would apply to the sum individual made up of everyone hired. This would produce the right truth conditions; it would imply that 75% of the individuals hired by the committee were women (assuming a context in which μ measures cardinality). But can a predicate undergo QR and leave a trace of type e?

The following case presents an even more vexing compositional challenge:

    1. (111)
    1. The manufacturers use 75% domestic tobacco.

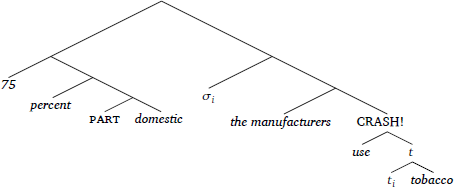
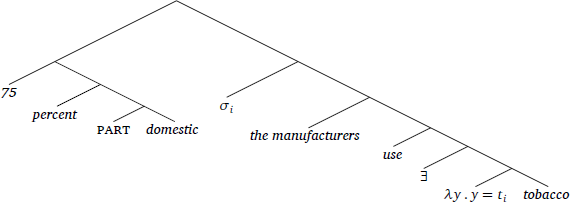
As discussed above, this seems to mean that 75% of the tobacco the manufacturers use is domestic. Pasternak & Sauerland (2022) would get that reading by placing focus on domestic. Within the current framework, and pursuing the idea that 75% domestic forms a unit that acts as a predicate, the same truth conditions could be obtained if it were somehow possible to extract that predicate and sum over the content contributed by the rest of the sentence. It’s not entirely trivial to do that, though, because if extracting 75% domestic from its position preceding tobacco left a trace of type e, then it wouldn’t be able to combine properly with its surrounding grammatical context:

    1. (112)

An LF that would yield the right result would look something like the following (modulo QR of the object):

    1. (113)

as if Partee’s (1987) ident type-shift had applied to the trace; ident(x) = λy . y = x.

In fact, it would be sensible to assume that the same kind of trace is left in (109), so we have:

    1. (114)

Both of these LFs could be achieved by a covert transformation rule like the following analogue to QR:

    1. (115)
    1. Predicate Raising

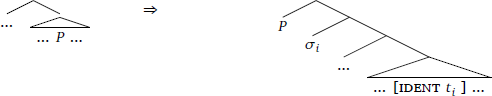
where P is type ⟨e, t⟩ and ident is a silent lexical item achieving the ident shift:

    1. (116)
    1. ident ⟿ λxλy . y = x

In the next section, I will suggest a way of implementing this basic idea in a dynamic framework using independently-motivated mechanisms developed to account for plural anaphora. I’ll also argue that these tools can also be used to shed light on the cumulative-like readings as in mix 10% manure with 90% coal.

6 Cumulative-like readings

The suggestion in the previous section does not suffice to account for cumulative-like readings, as in the mix example discussed above, repeated here:

    1. (117)
    1. They mix 10 percent manure with 90 percent coal.

This example could be accounted for fairly easily if the two percent-nominals were coordinated; we could then have a coordinated predicate that holds of x if 10% of x is manure and 90% of x is coal. But as argued above, it seems that the with phrase introduces its own argument of the verb, rather than being syntactically coordinated with the direct object.

A sum discourse referent can be introduced by the same mechanisms that produce plural anaphora in the following sentence:

    1. (118)
    1. Johnx went to the bakery with Maryy. Theyx+y bought a baguette.

In a plural dynamic logic such as Keshet’s (2019) Plural Update Semantics (PLUS), this could be represented as follows:

    1. (119)
    1. [x]; john(x); [y]; mary(y); gotobakerywith(x, y); [z]; baguette(z); buy(x ⊕ y)

where ⊕ denotes an ordinary sum operation over individuals. The semi-colons denote dynamic conjunction, and formulas consisting only of a discourse referent in brackets like [x] serve to introduce a new discourse referent.9

A first stab at a meaning representation for (117), then, might be the following:

    1. (120)
    1. [x]; [y]; mix(z, x, y); 10%(part(manure))(x ⊕ y); 90%(part(coal))(x ⊕ y)

Here I assume that 90% (part(coal)) denotes the predicate that our static system above derives for 90 percent part coal, with the part type-shift.

But these truth conditions are a bit too weak. All they say is that there is a sum x + y such that 10% of it was manure and 90% was coal (and x was mixed with y). They don’t require that 90% of what was mixed in, total, was coal. To enforce that, we can sum over all candidate values for x ⊕ y using Keshet’s + operator, which gives the sum over all candidate values for a given discourse referent. In PLUS, the only terms are variables, and variables prefixed by +, whose interpretation is the sum of all current candidate referents for the variable:

    1. (121)
    1. Interpretation of terms in PLUS
    2. • ||t||g,σ = g(t)
    3. • ||+t||g,σ = sum({g(t) : g ∈ σ})
    4. where g is an assignment, σ is a set of assignments (a state), and for any set S, sum(S) denotes the smallest plural individual that contains every member of S as a subpart.10

With this operator in hand, we can characterize the semantics of (117) as follows:10

    1. (122)
    1. [x]; [y]; mix(z, x, y); 10%(part(manure))(+(x ⊕ y)); 90%(part(coal))(+(x ⊕ y))

Now, 90% coal applies to the sum of all of the x + y sums such that x was mixed with y.11

This sum-operator provides an elegant representation of the meaning of the reversed cases as well. For example, it would allow us to represent They hired 75% women as follows:

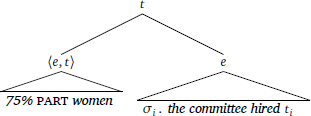
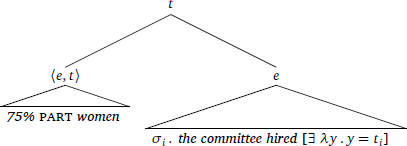
    1. (123)
    1. [x]; hired(z, x); 75%(part(women))(+x)

Here +x denotes the sum of all x such that they hired x. For They used 75% domestic tobacco, a natural representation would be:

    1. (124)
    1. [x]; use(z, x); tobacco(x); 75%(part(domestic))(+x)

Here +x denotes the sum of all x such that they used x and x is tobacco. I conjecture, therefore, that part-introducing percent might always apply to a cross-assignment sum, evaluated after the rest of the constraints in the clause have been applied to the discourse referent in question. I suspect, moreover, that there may be some connection between the use of a cross-assignment sum and the restricted set of verbs that give rise to reversed readings, but I must leave that connection unexplored in the present work. I leave it to future research to develop this in a compositional dynamic framework and thoroughly evaluate its predictions.

7 Conclusion

This paper has explored a wide range of part-introducing uses of percent, including not only the ‘reversed’ uses (as in The committee hired 75% women), but also predicative (as in The committee is 75% women), and attributive uses (as in They used 50% recycled paper). On the empirical side, this paper also showed that the range of ‘reversed’ uses is restricted to a limited set of verbs, and that certain cumulative-like examples can be found (as in They mix 10% manure with 90% coal).

In order to give a unified analysis of these part-introducing uses, I made two suggestions. First, I proposed a type-shift called part that converts a non-gradable predicate to a gradable one that tracks mereological parthood. This makes any non-gradable predicate eligible for use with Pasternak’s (2019) analysis of percent in constructions like 75% full. A slide modification of Pasternak’s (2019) analysis of percent provides an adequate analysis of the scalar predicative uses that can easily be extended to the scalar attributive uses. With the part operator, we gain an adequate treatment of the non-scalar, part-introducing predicative and attributive uses, as well as the reversed uses. Second, motivated in part by slight compositional challenges in the realm of reversed uses but mainly in order to account for cumulative-like readings, I sketched a dynamic theory based on Keshet’s (2019) PLural Update Semantics, in which plural discourse referents that summarize the accumulated constraints on a given discourse referent can serve as the ‘whole’ for a percent phrase that both introduces and serves as a predicate. While it is only a sketch, it promises to deliver an elegant account not only of cumulative-like readings but also of reversed uses.

This investigation has raised a number of questions that I hope will be addressed in future work, including: why the range of reversed uses is limited to a small class of verbs, how to capture the cumulative and reversed uses with a fully-explicit and unified compositional analysis, how to explain the infelicity of cumulative-like readings in scenarios like the one given in footnote 11, and how to analyze the wider range of uses of percent listed in the appendix.12 It seems that by making a small amount of progress, we have created much more work for ourselves.

Additional file

The additional file for this article can be found as follows:

Supplementary file

Corpus study. DOI: https://doi.org/10.16995/glossa.5791.s1

Abbreviations

LF = Logical Form, QR = Quantifier Raising, PLUS = Plural Update Semantics

Notes

  1. The original observation was made by Ahn (2012) for Korean, and subsequent work has focussed primarily on German and English (Sauerland 2014; Ahn & Sauerland 2015; 2017; Pasternak & Sauerland 2022; Li 2018; Solt 2018; Spathas 2019; Pasternak 2019). [^]
  2. The verb consists (of) is arguably not an ordinary transitive verb, expressing a relation between two individuals; it’s rather more like a copula, insofar as it links an individual with a characterization of that individual. I will return to this in &2.3. [^]
  3. Another interesting case that might fit into this category involves the verb divide, which doesn’t usually take adjectival complements but seems to in this example:
      1. (i)
      1. They counted 514 plain M&M’s; in a one-pound bag that divided 29 percent brown, 19 percent blue, 17 percent yellow, 15 percent orange, 12 percent red, and 8 percent green.
    [^]
  4. This was observed by Ezra Keshet (p.c.). [^]
  5. Pasternak & Sauerland (2022) write the context-sensitive μ function as μc, where c denotes the relevant context. I will not use a superscript c for μ in the representation language. Rather, I will assume that it is an indexical constant of the language like i, which picks out the speaker of the given context of utterance; just as ⟦i⟧M,g,c = the speaker of c, so ⟦μ⟧M,g,c depends on some feature of context c. This is a purely stylistic matter. [^]
  6. I’m using an indirect interpretation style here, which involves translating from English to a version of typed lambda calculus. It’s not entirely settled how to deal with focus in an indirect interpretation style, as far as I know. Here I’m assuming that the representation language imports a number of set-theoretic devices and that it contains a context-sensitive function alt that takes a natural language string or parse tree as an argument. [^]
  7. Note that the fraction appearing to the left of the equals sign in (89) involves division among degrees, implicitly presupposing a foundation for degree semantics that allows one degree to be divided by another, such as the system laid out by Coppock (2021). [^]
  8. I am grateful to a careful reviewer for pointing this out to me. [^]
  9. The semantics of formulas is given relative to a given input state σ:

    • σ⟦[x]⟧ = {h|∃g ∈ σ : g[x]h}

    • σ⟦ϕ;ψ⟧ = (σ⟦ϕ⟧)⟦ψ⟧

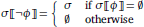
    • σ⟦P(t1,…,tn)⟧ = {g ∈ σ :⟨||t1||g,σ,…,||tn||g,σ⟩ ∈ ||P|}

    • σ⟦x=y⟧ = {g ∈ σ : g(x) = g(y)}

    • σ⟦[x=y]⟧ = (σ⟦[x]⟧)⟦x = y⟧

    [^]
  10. Keshet treats the denotation as the set itself, but treating the denotation as a plural individual works better for the purpose of giving a unified account of percent, as ‘NUM% ADJ’ can clearly function as a property of an individual, as in The glass is 75% full. The + operator is the crucial innovation in PLUS setting it apart from Dynamic Predicate Logic (Groenendijk & Stokhof 1991). [^]
  11. A reviewer points out that these truth conditions might be too weak. Suppose Jack has two barrels. In one barrel, there is a mixture weighing 50lbs consisting of 10lbs of manure thoroughly mixed with 40lbs of coal. The other barrel contains 50lbs of coal. Jack combines the contents of the two barrels. Did Jack mix 10% manure with 90% coal? The analysis in (122) would predict it is true in the situation described, but it doesn’t seem like an appropriate description. In future research, it would be worth probing whether the sentence is really false in this situation or merely pragmatically infelicitous. [^]
  12. Another open question is the relation between the cumulative cases and other somewhat similar cases like the following (Ezra Keshet, p.c.).
      1. (i)
      1. a.
      1. They mixed 1 part manure to 3 parts coal.
      1.  
      1. b.
      1. They mixed 1 quarter manure with 3 quarters coal.
    Note that part and quarter are not entirely analogous:
      1. (ii)
      1. a.
      1. #They mixed 1 part manure into their product.
      1.  
      1. b.
      1.   They mixed 1 quarter manure into their product.
    It seems that part is not interpretable without a specification of the total number of parts. [^]

Acknowledgements

I wish to thank the special collection editors Uli Sauerland and Robert Pasternak, along with two anonymous reviewers, for their careful and excellent feedback on this work.

Competing Interests

The author has no competing interests to declare.

References

Ahn, Dorothy. 2012. Reverse quantification in Korean. Term paper, Harvard University.

Ahn, Dorothy & Sauerland, Uli. 2015. The grammar of relative measurement. In Semantics and Linguistic Theory (SALT) 25, 125–142. DOI:  http://doi.org/10.3765/salt.v25i0.3062

Ahn, Dorothy & Sauerland, Uli. 2017. Measure constructions with relative measures: Towards a syntax of non-conservative construals. The Linguistic Review. DOI:  http://doi.org/10.1515/tlr-2017-0001

Beaver, David & Krahmer, Emiel. 2001. A partial account of presupposition projection. Journal of Logic, Language and Information 10. 147–182. DOI:  http://doi.org/10.1023/A:1008371413822

Champollion, Lucas. 2009. Cumulative readings of every do not provide evidence for events and thematic roles. In Proceedings of the nineteenth Amsterdam Colloquium. DOI:  http://doi.org/10.1007/978-3-642-14287-1_22

Coppock, Elizabeth. 2021. Challenge problems for a theory of degree multiplication. Proceedings of Semantics and Linguistic Theory (SALT) 31. DOI:  http://doi.org/10.3765/salt.v31i0.5071

Coppock, Elizabeth & Beaver, David. 2015. Definiteness and determinacy. Linguistics and Philosophy 38(5). 377–435. DOI:  http://doi.org/10.1007/s10988-015-9178-8

Erteschik-Shir, Nomi. 2007. Information structure. Oxford University Press.

Foley, William A. 2007. A typology of information packaging in the clause. In Shopen, T. (ed.), Language typology and syntactic description 1. 362–446. Cambridge: Cambridge University Press. DOI:  http://doi.org/10.1017/CBO9780511619427.007

Groenendijk, Jeroen & Stokhof, Martin. 1991. Dynamic predicate logic. Linguistics and Philosophy 14. 39–100. DOI:  http://doi.org/10.1007/BF00628304

JCGM. 2012. International vocabulary of metrology - basic and general concepts and associated terms (VIM), 3rd edition. Joint Committee for Guides in Metrology (JCGM).

Kennedy, Christopher & McNally, Louise. 2005. Scale structure, degree modification, and the semantics of gradable predicates. Language 81(2). 345–381. DOI:  http://doi.org/10.1353/lan.2005.0071

Keshet, Ezra. 2019. Discourse plurals in an update semantics. In Espinal, M. Teresa & Castroviejo, Elena & Leonetti, Manuel & McNally, Louise & Real-Puigdollers, Cristina (eds.), Proceedings of Sinn und Bedeutung 23. 557–571.

Krifka, Manfred. 1986. Nominalreferenz und Zeitkonstitution. Zur Semantik von Massentermen, Pluraltermen und Aspektklassen. Munich, Germany (published 1989): Fink.

Lambrecht, Knud. 1994. Information structure and sentence form: Topic, focus, and the mental representation of discourse referents. Cambridge: Cambridge University Press. DOI:  http://doi.org/10.1017/CBO9780511620607

Li, Haoze. 2018. Event-related relative measurement. In Truswell, Robert & Cummins, Chris & Heycock, Caroline & Rabern, Brian & Rohde, Hannah (eds.), Sinn und Bedeutung 21. 801–818. University of Konstanz.

Partee, Barbara H. 1987. Noun phrase interpretation and type-shifting principles. In Groenendijk, Jeroen & de Jongh, Dick & Stokhof, Martin (eds.), Studies in Discourse Representation Theory and the theory of generalized quantifiers, 115–143. Dordrecht: Foris. DOI:  http://doi.org/10.1515/9783112420027-006

Pasternak, Robert. 2019. Unifying partitive and adjective-modifying percent. Snippets 37. 77–79. DOI:  http://doi.org/10.7358/snip-2019-037-past

Pasternak, Robert & Sauerland, Uli. 2022. German measurement structures: Casemarking and non-conservativity. Accepted for publication in the Journal of Comparative Germanic Linguistics.

Raposo, Álvaro P. 2018. The algebraic structure of quantity calculus. Measurement Science Review 18(4). 147–157. DOI:  http://doi.org/10.1515/msr-2017-0021

Raposo, Álvaro P. 2019. The algebraic structure of quantity calculus II: Dimensional analysis and differential and integral calculus. Measurement Science Review 19(2). 70–78. DOI:  http://doi.org/10.2478/msr-2019-0012

Rett, Jessica. 2018. The semantics of many, much, few, and little. Language and Linguistics Compass 12. DOI:  http://doi.org/10.1111/lnc3.12269

Sauerland, Uli. 2014. Surface non-conservativity in German. In Piñon, Christopher (ed.), Empirical Issues in Syntax and Semantics 10, 125–142. http://www.cssp.cnrs.fr/eiss10/.

Scha, Remko. 1981. Distributive, collective and cumulative quantification. In Groenendijk, Jeroen & Janssen, Theo & Stokhof, Martin (eds.), Formal methods in the study of language, part 2, 483–513. Amsterdam: Mathematical Centre.

Schwarzschild, Roger. 2006. The role of dimensions in the syntax of noun phrases. Syntax 9(1). 67–110. DOI:  http://doi.org/10.1111/j.1467-9612.2006.00083.x

Solt, Stephanie. 2009. The semantics of adjectives of quantity. New York, NY: The City University of New York PhD dissertation.

Solt, Stephanie. 2018. Proportional comparatives and relative scales. In Truswell, Robert & Cummins, Chris & Heycock, Caroline & Rabern, Brian & Rohde, Hannah (eds.), Sinn und Bedeutung 21. 1123–1140.

Spathas, Giorgios. 2019. Plural anaphoric reference and non-conservativity. Snippets 37. 95–96. DOI:  http://doi.org/10.7358/snip-2019-037-spat

Szabolcsi, Anna. 1997. Ways of scope taking. Dordrecht: Kluwer. DOI:  http://doi.org/10.1007/978-94-011-5814-5

Wellwood, Alexis. 2015. On the semantics of comparison across categories. Linguistics and Philosophy 38(1). 67–101. DOI:  http://doi.org/10.1007/s10988-015-9165-0