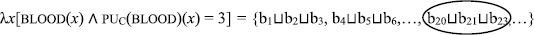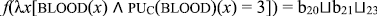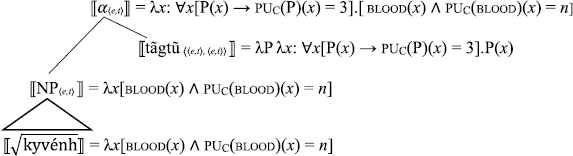1 Introduction
In this paper I examine the semantics of nominal countability in Kaingang, a Jê language spoken in southern Brazil. I argue that Kaingang parallels Yudja (Lima 2014a; b), a Tupi language, when it comes to countability in the nominal domain. Namely, in Kaingang both individual and substance nouns are interpreted as count nouns. This finding suggests that the generalized count interpretation of all nouns is not an idiosyncrasy of Yudja. However, contrary to Lima’s analysis (Lima 2014a; b) of Yudja, according to which the count meaning of Yudja nouns is derived via a covert morphological operation, my main hypothesis is that all Kaingang nouns are inherently count. This claim is based on the replication of various morphosyntactic and semantic tests found in the literature (Barner & Snedeker 2005; Bloomfield 1933; Doetjes 1997; Lima 2014a; b; Lima & Rothstein 2020; Pires de Oliveira & Rothstein 2011; Rothstein 2010; 2017). First, Kaingang allows numerals to combine directly with individual- and substance-denoting nouns. For substance nouns, even non-conventionalized portion-units can be counted. Second, count quantity expressions freely combine with both types of nouns. For example, the quantity words e ‘many’ and pipir ‘few’, whether forming a constituent with an individual or a substance noun, always trigger the cardinal interpretation of a large/small number of units. Third, bare substance nouns can also appear in constructions with count adjectives and be the antecedents of reciprocals. In addition, the application of Barner & Snedeker’s (2005) quantity judgement tasks shows that in comparative constructions with substance nouns Kaingang speakers not only access cardinality-based readings, but they also consistently privilege them. That is, their default evaluation is based on cardinality.
I analyze this pervasive counting strategy in Kaingang as a direct effect of the lexical meaning of root nouns in the language. Drawing on Krifka’s approach (1989; 2007; 2008), my hypothesis is that all nouns come from the lexicon already equipped with a context-sensitive built-in counting function that, depending upon the type of noun, measures quantities in terms of individual or portion-units. This amounts to saying that all nouns in Kaingang are born quantized.
This paper is structured as follows. In section 2, I provide some information about Kaingang bare nouns that is relevant for a better understanding of the data and make some comments on methodology. In section 3, I present the data supporting the main hypothesis of this paper. Section 4 contains my analysis of the generalized count meaning of Kaingang nouns. First, in subsection 4.1, I discuss in broad lines the theoretical approach my analysis builds on, namely Krifka’s theory of countability (1989; 2007; 2008). Subsequently, in subsection 4.2, I provide the analysis. I treat all nouns in Kaingang as lexically quantized due to a context-sensitive quantizing function incorporated into their root denotations, which provide the units for counting. I argue that such an analysis is not only supported by the pervasive count interpretation of Kaingang nouns, but also motivated by crosslinguistic evidence. In the same section, I show that a treatment of count nouns as quantized predicates with a built-in measure function à la Krifka’s nu (natural-unit) is compatible with modeling them as being of type ⟨e,t⟩, rather than a relation between numbers and sets, as in Krifka’s work. With this solution, I intend to show that Kaingang bare nouns can be used to refer to pluralities picked out from the denotation of quantized nouns, which means that plural readings of bare nouns uninflected for number don’t need a cumulative reference at the n(p) or nump level. In section 5, I briefly discuss Lima’s (2014a; b) analysis of Yudja. There I hope to show that analysing Kaingang and Yudja count nouns as quantized predicates, rather than (derived) atomic cumulative ones, as Lima advocates for Yudja, has the explanatory advantage of allowing a crosslinguistic generalization about the semantics of count nouns. In section 6, I deal with one potential challenge to my analysis. This challenge comes from a version of the quantity judgement test: one in which two individual- or portion-units, differing in volume, are compared. The initial explanation that suggests itself is that speakers allow evaluations along the volume dimension in these scenarios. However, I propose a reinterpretation of these data which makes them compatible with a cardinality judgement. In section 7, I highlight some contributions of the findings in this paper for the study of crosslinguistic variations in the expression of nominal countability. Finally, in section 8 I offer my conclusions.
2 The language
Kaingang is a Jê language spoken in Southern Brazil by the Kaingang people. The Kaingang population is estimated to be 37,400 people, mostly living in Indigenous territories in four states: São Paulo, Paraná, Santa Catarina, and Rio Grande do Sul. Currently, Kaingang is estimated to be spoken by 22,000 people (Instituto Brasileiro de Geografia e Estatística 2012). The current orthographic system of Kaingang was developed between 1958 and 1966 by Ursula Wiesemann and a group of native speakers of the language. This joint work also culminated in a Kaingang-Portuguese dictionary (Wiesemann 1971), which decades later received an updated version (Wiesemann 2002).
The data in this paper originated from two periods of fieldwork I conducted in the Kaingang territory of Rio das Cobras, in the state of Paraná, Brazil. They were collected through direct elicitation with three native speakers, aged 25, 43, and 46 years old. In each elicitation session speakers were asked questions designed to prompt answers that rely on their linguistic knowledge of Kaingang. The data were collected through a set of standard elicitation procedures: (i) grammaticality judgments, (ii) translations, and (iii) pairing of sentences with discourse contexts, in the form of verbal descriptions, or through language-independent single images (and storyboards) (Barner & Snedeker 2005; Bochnak & Matthewson 2020; Bohnemeyer 2015; Krifka 2011; Matthewson 2004; 2012). As for procedure (iii), it consisted of setting up discourse contexts and asking the consultants whether sentences are felicitous in them. This strategy relies on either elicited truth-value or felicity/acceptability judgments.
Kaingang is a generalized bare noun language, i.e., nouns can occur in all argument positions without any functional material, such as articles or number inflection, as illustrated in (1–3).
- (1)
- Gĩr
- child
- vỹ
- nom
- jãn
- sing
- ∅.1
- pfv
- ‘A/some/the child(ren) sang.’
- (2)
- Penkỹ
- bowl
- gãm ke
- break
- ∅.
- pfv
- ‘A/some/the bowl(s) broke.’
- (3)
- Gĩr
- child
- vỹ
- nom
- ka
- tree
- vé
- see
- ∅.
- pfv
- ‘A/some/the child(ren) saw a/some/the tree(s).’
The canonical word order in Kaingang is sov, as in (3). As the translations above indicate, Kaingang bare nouns can have indefinite and definite interpretations. Both readings are observed in the ability of bare nouns to introduce novel discourse referents and anaphorically refer to them, as shown in (4).
- (4)
- Rãkétá
- yesterday
- gĩr1
- child
- vỹ
- nom
- mur
- born
- ∅
- pfv
- jamã
- village
- ki.
- in
- ‘Yesterday a child was born in the village.’
- Gĩr1
- child
- tóg
- top
- mág
- big
- nĩ.
- asp
- ‘The child is big.’
Further evidence of indefinite readings of bare nouns comes from the conjunction of a sentence and its negation, which doesn’t give rise to a contradiction in contexts with non-unique referents. This fact is illustrated in (5).
- (5)
- Context: You made two chairs. Yesterday one of them broke and the other didn’t.
- Nĩgja
- chair
- mráj
- break
- ∅,
- pfv
- hãra
- but
- nĩgja
- chair
- mráj
- break
- ∅
- pfv
- tũ
- neg
- nĩ.
- asp
- ‘A chair broke, but a chair didn’t break.’
Kaingang bare nouns are unmarked for number and can be used to refer to singularities or pluralities, i.e., they are number neutral. For instance, the bare noun fóg ‘non-indigenous person’ in (6) can be the antecedent to either singular or plural pronouns.
- (6)
- Fóg1
- non_indigenous_person
- vỹ
- nom
- ẽg
- 1poss.pl
- jamã
- village
- mĩ
- inside
- kãtĩg
- come
- ∅.
- pfv
- ‘A/some/the non-indigenous person(s)1 came to our village.’
- Fi/fag1
- 3sg.f/3pl.f
- tóg
- top
- pã′i
- chief
- ĩn
- house
- tá
- there
- nũr
- sleep
- ∅.
- pfv
- ‘She/they1 slept in the chief’s house.’
Because bare nouns are underspecified for number, they are compatible with collective predicates, as in (7), and provide the discourse set for (multiple) indefinites in follow up clauses, as in (8).
- (7)
- Gĩr
- child
- vỹ
- nom
- vẽnh mãn
- get_together
- ∅.
- pfv
- ‘Some/the children got together.’
- (8)
- Gĩr
- child
- vỹ
- asp
- jun
- arrive
- ∅.
- pfv
- ‘Some/the children arrived.’
- Ũ
- indef
- vỹ
- nom
- téj
- tall
- jẽ
- asp
- jãvo
- and
- ũ
- indef
- vỹ
- nom
- rur
- short
- jẽ.
- asp
- ‘One is tall and one is short.’
The constructions above, which illustrate the unrestricted distribution of argument bare nouns, their definite and indefinite interpretations, as well as number neutrality, are sufficient to equip the reader with the information required to interpret the data in section 3.
3 Concrete units all over the place
3.1 Numerals
Kaingang is a non-classifier language, i.e., numerals can combine with individual-denoting nouns without any intervening classifier, as illustrated in (9) and (10).
- (9)
- Gĩr
- child
- régre
- two
- vỹ
- nom
- jun~jun
- red~arrive
- ∅.
- pfv
- ‘Two children arrived.’
- (10)
- Fógtẽ
- Fógtẽ
- vỹ
- nom
- gĩr
- child
- tãgtũ
- three
- vé
- see
- ∅.
- pfv
- ‘Fógtẽ saw three children.’
Like in familiar non-classifier languages, Kaingang numerals are compatible with substance nouns when the latter are coerced to refer to conventional packages, as in (11), or in subkind/taxonomic interpretations, as in (12).
- (11)
- Fógtẽ
- Fógtẽ
- vỹ
- nom
- goj
- water
- tãgtũ
- three
- kron
- drink
- ∅.
- pfv
- ‘Fógtẽ drank three (bottles of) water.’
- (12)
- Context: You tell a doctor what the blood types of your family members are.
- Ẽg
- 1poss.pl
- ĩn
- house
- tá
- there
- ẽg
- 1pl
- vỹ
- nom
- kyvénh
- blood
- régre
- two
- nỹtĩ:
- asp:
- A
- A
- kar
- then
- O.
- O
- ‘In our house we have two (kinds of) blood: A and O.’
Nevertheless, Kaingang departures from familiar non-classifier languages by allowing numerals to combine directly with substance nouns even when they are interpreted as referring to non-conventionalized portion-units. This is laid out in (13–16).
- (13)
- Context: You enter the kitchen and notice that there are three puddles of water on the floor.
- Ẽgnénh jãfã
- kitchen
- ki
- in
- tóg
- top
- goj
- water
- tãgtũ
- three
- nĩ.
- asp
- ‘There are three (puddles of) water in the kitchen.’
- (14)
- (Adapted from Lima 2014a)
- Context: On the way home from school Fógtẽ’s nose dripped three drops of blood.
- Kyvénh
- blood
- tãgtũ
- three
- vỹ
- nom
- nãg ke
- drip
- ∅.
- pfv
- ‘Three (drops of) blood dripped.’
- (15)
- Context: Fógtẽ saw three puddles of blood on the floor.
- Fógtẽ
- Fógtẽ
- vỹ
- nom
- kyvénh
- blood
- tãgtũ
- three
- vé
- see
- ∅.
- pfv
- ‘Fógtẽ saw three (puddles of) blood.’
- (16)
- (Adapted from Lima 2014a)
- Context: Pakój took a bucket full of sand from her house to Maria’s. On the way she spilled three portions of sand on the floor.
- Pakój
- Pakój
- vỹ
- nom
- rỹnhrỹj
- sand
- tãgtũ
- three
- kujẽn
- spill
- ∅.
- pfv
- ‘Pakój spilt three (portions of) sand.’
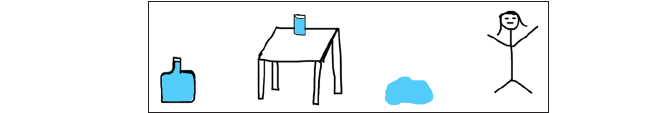
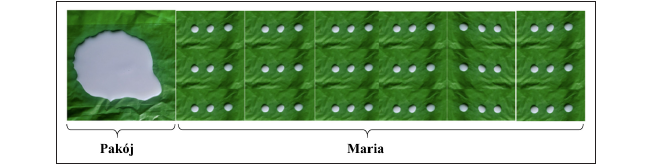
In (13), the counted portions are puddles of water. In (14) and (15) the units are by drops and puddles of blood, respectively, while in (16) they constitute portions of sand. In all cases the portions are contextually supplied and don’t correspond to conventionalized units. Furthermore, the portions counted in a situation can vary in shape, size, and containers, as in Context A (17), depicted in Figure 1. Together with the possibility of directly counting (non-)conventional units, (17) indicates that the ‘unitizing’ semantics of Kaingang nouns should be extremely general.2
- (17)
In sum, the data above show that in Kaingang numerals directly modify individual and substance nouns. The availability of substance noun+numeral constructions in contexts that supply non-conventional portions constitutes one piece of evidence that substance nouns in Kaingang can be interpreted as referring to individuated portions.
3.2 Quantity words
In many mass/count languages there are quantity words which select for particular types of nouns. For instance, in English, (a) little, less and much combine only with mass nouns, whereas (a) few, many, and several require count nouns. The availability of this distinction in the distribution of quantity words is often used as one diagnostic test for the existence of the mass/count distinction in a language (Bloomfield 1933; Doetjes 1997; 2012).
Kaingang has at least five quantity words: kar ‘all’, ũ ‘some’ e ‘many’, pipir ‘few’, and mẽ ‘more’. All these terms are compatible with individual and substance nouns, as illustrated by the minimal pairs in (18–22), where the quantity words immediately follow the nouns.
- (18)
- a.
- Gĩr
- child
- kar/ũ
- all/indef
- vỹ
- nom
- jãn~jãn
- red~sing
- ∅.
- pfv
- ‘All/some child(ren) sang.’
- b.
- Goj
- water
- kar/ũ
- all/indef
- vỹ
- nom
- ror
- round
- nỹtĩ.
- asp
- ‘All/some (puddle(s) of) water are round.’
- (19)
- a.
- Fógtẽ
- Fógtẽ
- vỹ
- nom
- gĩr
- child
- kar/ũ
- all/indef
- vé
- see
- ∅.
- pfv
- ‘Fógtẽ saw all/some child(ren).’
- b.
- Fógtẽ
- Fógtẽ
- vỹ
- nom
- kyvénh
- blood
- kar/ũ
- all/indef
- vé
- see
- ∅.
- pfv
- ‘Fógtẽ saw all/some (drop(s) of) blood.’
- (20)
- a.
- Gĩr
- child
- e/pipir
- many/few
- vỹ
- nom
- jãn~jãn
- red~sing
- ∅.
- pfv
- ‘Many/few children sang.’
- b.
- Goj
- water
- e/pipir
- many/few
- vỹ
- nom
- ror
- round
- nỹtĩ.
- asp
- ‘Many/few (puddles of) water are round.’
- (21)
- a.
- Fógtẽ
- Fógtẽ
- vỹ
- nom
- gĩr
- child
- e/pipir
- many/few
- vé
- see
- ∅.
- pfv
- ‘Fógtẽ saw many/few children.’
- b.
- Fógtẽ
- Fógtẽ
- vỹ
- nom
- kyvénh
- blood
- e/pipir
- many/few
- vé
- see
- ∅.
- pfv
- ‘Fógtẽ saw many/few (drops of) blood.’
- (22)
- a.
- Fógtẽ
- Fógtẽ
- vỹ
- nom
- gĩr
- child
- mẽ
- more
- nĩ
- ASP
- Pedro
- Pedro
- ve kỹ.
- than
- ‘Fógtẽ has more children than Pedro.’
- b.
- Fógtẽ
- Fógtẽ
- vỹ
- nom
- kyvénh
- blood
- mẽ
- more
- nĩ
- ASP
- Pedro
- Pedro
- ve kỹ.
- than
- ‘Fógtẽ has more blood than Pedro.’
The quantity expression e ‘many’ always preserves its cardinal interpretation of a large number of units, irrespective of the noun it combines with. When combined with a substance noun the large number will be of portions, as exemplified in (23). The infelicity of the sentence in (23) in context B results from the fact that this scenario doesn’t provide multiple portion-units for e to quantify over.
- (23)
- ✔Context A: There were many puddles of blood in front of a building. Fógtẽ saw them.
- ✖Context B: There was a large puddle of blood in front of a building. Fógtẽ saw it.
- Fógtẽ
- Fógtẽ
- vỹ
- nom
- kyvénh
- blood
- e
- many
- vé
- see
- ∅.
- pfv
- ‘Fógtẽ saw many (puddles of) blood.’
To describe a context like B, the adjective mág ‘big’ is used instead.
- (24)
- Fógtẽ
- Fógtẽ
- vỹ
- nom
- kyvénh
- blood
- mág
- big
- vé
- see
- ∅.
- pfv
- ‘Fógtẽ saw a/some/the big (puddle(s) of) blood.’
Note that due to the number neutrality of the noun kyvénh, (24) can also mean that Fógtẽ saw more than one big puddle of blood. As expected, the adjective mág isn’t in complementary distribution with e. Both can occur in the same nominal construction, always triggering the meaning of a large number of big individuals or portions:
- (25)
- Fógtẽ
- Fógtẽ
- vỹ
- nom
- gĩr
- child
- mág
- big
- e
- many
- vé
- see
- ∅.
- pfv
- ‘Fógtẽ saw many big children.’
- (26)
- Fógtẽ
- Fógtẽ
- vỹ
- nom
- kyvénh
- blood
- mág
- big
- e
- many
- vé
- see
- ∅.
- pfv
- ‘Fógtẽ saw many big (puddles of) blood.’
The same pattern is displayed by pipir ‘few’. Whether it combines with individual nouns, as in (27), or with substance nouns, as in (28) and (29), it consistently means a small number of individuated units.
- (27)
- Gĩr
- child
- pipir
- few
- vỹ
- nom
- jun~jun
- red~arrive
- ∅.
- pfv
- ‘Few children arrived.’
- (28)
- ✔Context A: Fógtẽ accidently cut his left foot and a few drops of blood dripped.
- ✖Context B: Fógtẽ accidently cut his left foot and one single drop of blood dripped.
- Kyvénh
- blood
- pipir
- few
- vỹ
- nom
- nãg ke
- drip
- ∅.
- pfv
- ‘Few (drops of) blood dripped.’
- (29)
- ✔Context A: There are two puddles of blood in front of a building. Fógtẽ saw them.
- ✖Context B: There is a single puddle of blood in front of a building. Fógtẽ saw it.
- Fógtẽ
- Fógtẽ
- vỹ
- nom
- kyvénh
- blood
- pipir
- few
- vé
- see
- ∅.
- pfv
- ‘Fógtẽ saw few (puddles of) blood.’
To describe a situation where a small portion of blood dripped, the count adjective sĩ ‘small’ is used instead.
- (30)
- Kyvénh
- blood
- sĩ
- small
- vỹ
- nom
- nãg ke
- drip
- ∅.
- pfv
- ‘A/some/the small (drop(s) of) blood dripped.’
The sentence in (30) can also be used to describe a situation with small units of blood, due to the number neutrality of the noun. Like e ‘many’ and mág ‘big’, pipir is not in complementary distribution with sĩ. Pipir can participate in a noun phrase headed by a noun already modified by sĩ, as in (31) and (32).
- (31)
- Gĩr
- child
- sĩ
- small
- pippir
- few
- vỹ
- nom
- jun~jun
- red~arrive
- ∅.
- pfv
- ‘Few small children arrived.’
- (32)
- Kyvénh
- blood
- sĩ
- small
- pipir
- few
- vỹ
- nom
- nãg ke
- drip
- ∅.
- pfv
- ‘Few small (drops of) blood dripped.’
Summarizing, the data in this subsection demonstrate that quantity words in Kaingang are compatible with individual and substance nouns, triggering the same interpretation with both types of nouns. Particularly, they show that the quantity terms e ‘many’ and pipir ‘few’ are associated with a cardinality interpretation even when combined with substance nouns.
3.3 Count adjectives and reciprocals
As already foreshadowed in subsection 3.2, substance nouns can occur with adjectives which hold of each singularity in the extension of a noun. In other words, Kaingang substance nouns can also be modified by adjectives which denote properties of individuated entities, such as mág ‘big’, sĩ ‘small’, and ror ‘round’. Because these adjectives mostly select for count nouns, Deal (2017) refers to them as ‘count adjectives’, whereas Schwarzschild (2011) calls them ‘stubbornly distributive adjectives’. Consider the minimal pairs below:
- (33)
- a.
- nĩgja
- chair
- mág
- big
- ‘big chair(s)’
- b.
- kyvénh
- blood
- mág
- big
- ‘big (portion(s) of) blood.’
- (34)
- a.
- nĩgja
- chair
- sĩ
- small
- ‘small chair(s)’
- b.
- kyvénh
- blood
- sĩ
- small
- ‘small (portion(s) of) blood.’
- (35)
- a.
- nĩgja
- chair
- ror
- round
- ‘round chair(s)’
- b.
- kyvénh
- blood
- ror
- round
- ‘round (portion(s) of) blood.’
In addition, these adjectives can also appear as predicative adjectives in constructions whose subjects are bare substance nouns:
- (36)
- Context: You notice that some drops of blood on the floor are round.
- Kyvénh
- blood
- tóg
- top
- ror
- round
- nỹtĩ.
- asp
- ‘Some (drops of) blood are round.’
- (37)
- Context: You notice that some drops of blood on the floor are big.
- Kyvénh
- blood
- tóg
- top
- mág
- big
- nỹtĩ.
- asp
- ‘Some (drops of) blood are big.’
In (36) and (37), the properties of being round and big distribute to each portion-unit of blood referred to by the bare noun.
Furthermore, like individual-denoting bare nouns, as in (38), bare substance nouns can be the antecedents for reciprocals, as in (39–41).
- (38)
- Gĩr
- child
- vỹ
- nom
- jagnẽ
- recp
- hã
- similar
- nĩ.
- asp
- ‘Some/the children look like each other.’
- (39)
- Context: After the rain there were separate puddles of water on the floor. You notice that some of them are similar in shape and size.
- Goj
- water
- tóg
- top
- jagnẽ
- recp
- hã
- similar
- nỹtĩ.
- asp
- ‘Some (puddles of) water look like each other.’
- (40)
- Context: A clay bench has cracked into three pieces. You notice that they are similar in shape and size.
- Óré
- clay
- tóg
- top
- jagnẽ
- recp
- hã
- similar
- nỹtĩ.
- asp
- ‘The (pieces of) clay look like each other.’
- (41)
- Context: There are three piles of flour on the table. You notice that they are similar in shape and size.
- Vẽnh kuri
- flour
- tóg
- top
- mesa
- table
- kri
- on
- jagnẽ
- recp
- hã
- similar
- nỹtĩ.
- asp
- ‘The (piles of) flour on the table look like each other.’
The constructions with substance nouns above involve the application of their reciprocal vps to a plurality containing unitized portions of a substance, each of which bears the reciprocal relation of being similar to all the others. The felicity of (39–41) shows that their subject bare nouns are interpreted as denoting sets of contextually salient portion-units of water, clay, and flour, respectively.
3.5 Quantity comparisons
3.5.1 Mẽ ‘more’
In languages that encode the mass/count distinction, count nouns are primarily compared based on cardinality, whereas substance mass nouns are evaluated based on volume/weight (Barner & Snedeker 2005). In English, for instance, the comparative sentence (42a) asserts that the cardinality of Pedro’s children is greater than the cardinality of Roberta’s children. In contrast, sentence (42b) means that the overall volume of flour that Roberta has is greater than the overall volume of flour that Pedro has.
- (42)
- a.
- Pedro has more children than Roberta.
- b.
- Roberta has more flour than Pedro.
Accordingly, depending on the noun replacing N in comparative constructions of the type X has more N than Y, speakers will privilege judgements along the number or volume dimensions.
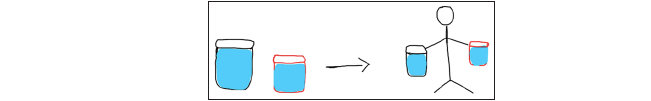
The Kaingang data laid out so far support the thesis that all nouns can be interpreted as count nouns and as such denote sets of counting units. This gives rise to the prediction that speakers will privilege number-based interpretations of all nouns in comparative constructions. To test this prediction, Barner & Snedeker’s (2005) quantity judgement tasks were applied. The consultants were shown pictures of real-world objects and substances. Each picture contained a collection of one or two objects or portions of a substance side-by-side with another collection of multiple objects of the same category or portions of the same substance. The quantities on the left side of the pictures were presented as belonging to one person and those on the right side to another one. Importantly, the total volume of the objects/portions on the left always exceeded those on the right, whereas the objects/portions on the right outnumbered those on the left. After showing these stimuli pictures to each consultant, they were asked the target comparative question in (43a) containing the quantity expression mẽ ‘more’, where N was replaced by an individual or substance noun, and then requested to provide one of the two possible answers in (43b).
- (43)
- a.
- Ũ
- indef
- nỹ
- q
- tỹ
- sbj
- N
- N
- mẽ
- more
- nĩ?
- asp
- ‘Who has more N?’
- b.
- Pakój/Maria
- Pakój/Maria
- vỹ
- nom
- N
- N
- mẽ
- more
- nĩ
- asp
- Maria/Pakój
- Maria/Pakój
- ve kỹ.
- than
- ‘Pakój/Maria has more N than Maria/Pakój.’
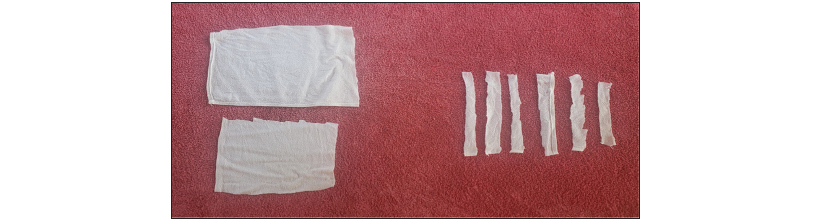
Stimuli were designed with six nouns of each type, as listed in (44). The pictures were presented in a random order.
- (44)
- Individual-denoting nouns:
- Substance-denoting nouns:
- gĩr ‘child’, kasor ‘dog’, ka ‘tree’, kakanẽ ‘fruit’, kur
- ‘clothes/clothing’, nĩgja ‘bench/chair/stool’.
- goj ‘water’, kyvénh ‘blood’, rénhte ‘milk’, venh kuri ‘flour’,
- kur ‘fabric’,3 vãfe ‘string’
Note that although the nouns kur ‘fabric’ and vãfe ‘string’ do not denote substances, I heuristically include them in the substance nouns category because cross-linguistically they are often treated as mass nouns compared in terms of volume/size (Deal 2017). Figures 2, 3, 4, 5, 6, 7 display six instances of the visual stimuli provided in the comparative tasks.
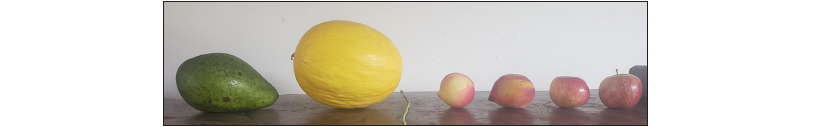
In accordance with the prediction, speakers systematically compared all nouns along the number dimension. Namely, even in scenarios containing substances, like in Figure 2, and a target question like (45), there were no volume-based interpretations.
- (45)
- Ũ
- indef
- nỹ
- q
- tỹ
- sbj
- rénhte
- milk
- mẽ
- more
- nĩ?
- asp
- ‘Who has more milk?’
Although the volume of the portion of milk on the left of Figure 2 is larger than the total volume of the three drops on the right, the speakers without hesitation counted the number of portions. That is, in a context where the portion of milk on the left edge belongs to Pakój, while all the other portions to Maria, the three consultants, when asked (45), responded that Maria had more milk. As a result, these findings offer further supporting evidence that all nouns in Kaingang are interpreted as count nouns.
3.5.2 E ‘many’
Given the count meaning of e ‘many’, particularly when it combines with substance nouns, one immediate prediction is that when exposed to the same battery of visual stimuli exemplified in Figures 2, 3, 4, 5, 6, 7 and then asked the question ‘(46a)’, speakers will offer a cardinality-based judgement. Thus, to test this, the consultants were shown most of the pictures used along with the sentences in (43). In this task, after having been shown a picture exhibiting on its left side a collection of one or two objects/portions and on its right side three or more objects/portions, the consultants were exposed to the target sentences in (46).
- (46)
- a.
- Ũ
- indef
- nỹ
- q
- N
- N
- e
- many
- nĩ?
- asp
- ‘Who has many N?’
- b.
- Pakój/Maria
- Pakój/Maria
- vỹ
- nom
- N
- N
- e
- many
- nĩ.
- asp
- ‘Pakój/Maria has many N.’
Once more the consultants’ responses were as predicted, i.e., they systematically associated e with a cardinality interpretation, whether it applies to individual or substance nouns. A volume-based evaluation didn’t occur. Hence, this finding adds, evidence in support of the main hypothesis of this study.
3.5.3 Mág ‘big’
If substance nouns denote sets of portion-units and mág ‘big’ is a count predicate, it falls out naturally that in quantity tasks mág will be interpreted as a property assigned to individual- or portion-units. This diagnostic test was first proposed by Lima (2014a; b), as part of her study of countability in Yudja. To investigate this prediction, I again used the number neutral nouns in (44). The visual stimuli were constituted by pictures containing on the left side one big object or portion of a substance and on the right side multiple objects of the same category or portions of the same substance. Below is the question employed, followed by the two opposing responses.
- (47)
- a.
- Ũ
- indef
- nỹ
- q
- N
- N
- mág
- big
- nĩ?
- asp
- ‘Who has a big N?’
- b.
- Pakój/Maria
- Pakój/Maria
- vỹ
- nom
- N
- N
- mág
- big
- nĩ.
- asp
- ‘Pakój/Maria has a big N.’
For illustration, I lay out in Figures 8, 9, 10, 11 four samples of the pictures used as stimuli.
The prediction that mág primarily would be attributed to the single object or portion on the left side of each picture was confirmed. Therefore, this result offers further evidence in support of the thesis that, like individual nouns, substance nouns in Kaingang provide units for counting. So much so that even in a scenario where the total volume of the multiple portions of a substance exceeds the volume of the single portion on the left edge of the picture, speakers compared the sizes of individuated portions, not their overall volume. For instance, in a situation where the portion of milk on the left edge belongs to Pakój, while all the other portions to Maria, as displayed in Figure 12, the default response of the consultants, when asked (47a), was that Pakój had a big unit of milk.
3.6 A brief note on container words
Kaingang allows for the optional use of container phrases in constructions with numerals and substance nouns. In such configurations container words like runja ‘bucket’ and kej ‘basket’ are complements of a locative, like in Yudja (Lima 2014; 2016), or of a postposition of instrument. For example, the container noun runja ‘bucket’ in (48c) is the complement of the same postpositions as in (48a–b).
- (48)
- a.
- Ĩn
- house
- ki
- inside
- gĩr
- child
- tóg
- top
- nỹtĩ.
- asp
- ‘A/some/the child(ren) is/are in a/some/the house(s).’
- b.
- Fógtẽ
- Fógtẽ
- vỹ
- nom
- nĩgja
- chair
- mraj
- break
- ∅
- pfv
- ka
- stick
- tỹ.
- with
- ‘Fógtẽ broke a/some/the chair(s) with a/some/the stick(s).’
- c.
- Fógtẽ
- Fógtẽ
- vỹ
- nom
- runja
- bucket
- ki/tỹ
- inside/with
- rỹnhrỹj
- sand
- régre
- two
- tatĩn
- carry
- ∅.
- pfv
- ‘Fógtẽ carried two (portions of) sand in/with a/some/the bucket(s).’
Note that in (48c) the container phrase runja ki/tỹ ‘in/with bucket(s)’ doesn’t occur between the numeral régre ‘two’ and the substance noun rỹnhrỹj ‘sand’. A construction in which the container phrase intervenes between the numeral and the noun is ruled out in Kaingang, as illustrated in (49).
- (49)
- ??Fógtẽ
- Fógtẽ
- vỹ
- nom
- goj
- water
- runja
- bucket
- ki/tỹ
- inside/with
- régre
- two
- tatĩn
- carry
- ∅.
- pfv
- ‘Fógtẽ carried two buckets of water.’
Thus, the syntactic configuration in (48c) and the consultants’ rejection of (49) suggest that container phrases in Kaingang are adjuncts.
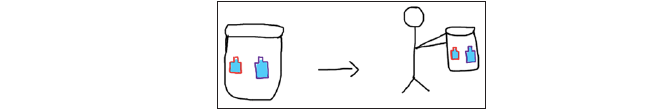
Like in English, in Kaingang and Yudja container phrases can be interpreted as providing the units for counting. However, as opposed to English, in Kaingang and Yudja container phrases can also have a locative meaning. That is, sentences like (48c) can be interpreted as referring to locations where already individuated units are placed.4 Consider sentence (50) and the three contexts below depicted in Figures 13, 14, 15.
- (50)
- Fógtẽ
- Fógtẽ
- vỹ
- nom
- runja
- bucket
- ki/tỹ
- inside/with
- goj
- water
- régre
- two
- tatĩn
- carry
- ∅.
- pfv
- ‘Fógtẽ carried two (portions of) water inside/with a/some/the bucket(s).’
- ✔Context A: Fógtẽ carried two buckets with water.
- ✔Context B: Fógtẽ poured two bottles of water in a bucket and carried it.
- ✔Context C: Fógtẽ put two closed bottles full of water in a bucket and carried it.
The construction in (50) is felicitous in all the three scenarios above. One crucial element common to all of them is that the quantity of water carried can be mapped onto two concrete portions of water. In context A, the two portions are individuated by two buckets, i.e., units and buckets coincide. In context B, although the quantity of water carried isn’t individuated inside the bucket, it can be traced back in time to two concrete bottles that partitioned the quantity into two counting units. As for context C, in it the container phrase is interpreted as just indicating the receptacle where the concrete counting units individuated by the two bottles are placed, i.e., the bucket is neither used to individuate nor to measure the portions.
The felicity of the sentence in (50) in context c, hence, shows that Kaingang container phrases can also have a locative interpretation, i.e., one that indicates where concrete counting units are located. Thus, the fact that Kaingang container phrases are adjuncts, that they cannot intervene between numerals and (substance) nouns, as well as the fact that the interpretation of constructions like the one in (50) doesn’t always associate the units with the receptacles denoted by the container nouns, are quite compatible with an analysis of Kaingang substance nouns as count nouns that denote portion-units.
Summarizing, this section provided a list of morphosyntactic and semantic pieces of evidence showing that individual and substance nouns in Kaingang pattern alike in the counting system of the language, i.e., there are no morphosyntactic or semantic features that distinguish them in this domain. These findings support the hypothesis that all nouns in Kaingang are interpreted as count. Like individual nouns, substance nouns denote counting units not only when combined with numerals and other quantity words, or in comparative constructions, but also when they appear bare, as evidenced in the examples with reciprocals and count adjectives. As a result, the individuating mechanism involved in the count interpretation of Kaingang nouns cannot be attributed to numerals and quantity expressions. The only thesis that seems to be borne out by the facts is that the operation supplying the individual- or portion-units must already be encoded in the nouns themselves. The conclusion is that all nouns are inherently count in Kaingang, i.e., they enter derivations already vested with a count meaning. In section 4, I provide a semantic analysis of this lexical property shared by all Kaingang nouns.
4 The analysis
4.1 Theoretical background: Krifka’s approach
Krifka (1989; 2007; 2008) claims that the mass/count distinction is grounded in two semantic properties of predicates: quantization and cumulativity. A predicate P is quantized if and only if whenever P applies to both x and y, y cannot be a proper part of x, as formally stated in (51). In contrast, a predicate P is cumulative if and only if whenever it holds of two entities it also holds of their sum, as defined in (52).
- (51)
- (Krifka 1989: 78)
- quant(P) ⟺ ∀x∀y[[P(x) ∧ P(y)] → ¬(y ⊏ x)]
- (52)
- (Krifka 1989: 78)
- cum(P) ⟺ ∀x∀y[[P(x) ∧ P(y)] → P(x⊔y)]
Prototypical examples of a quantized predicate in mass/count languages such as English are constructions formed by the combination of numerals with count nouns. The constituent one child, for example, expresses a quantized predicate that is true of each individual child, but not of their sums. As for cumulative predicates, their paradigmatic instances are substance mass nouns like blood, which denote a set closed under sum formation, i.e., a join-semilattice structure.5 Crucially, (51) and (52) are mutually exclusive.
Count nouns are modeled by Krifka as two-place predicates which contain a measure function defined on a domain of entities structured by a complete join-semilattice. In this approach, the “built-in modes of dividing reference” (Quine 1960: 91) encoded by count nouns are accomplished by an additive measure function from entities to numbers (Krifka 1989), which provides a unit of measurement. Additive measure functions can also be expressed by classifiers or measure terms such as head-of, kilo-of, litter-of, etc. Formally, Krifka defines an additive measure function as follows:
- (53)
- (Adapted from Krifka 2008: 2)
- μ is an additive measure function with respect to ⊔ iff μ maps entities to numbers such that: ¬ x∘y → [μ(x⊔y) = μ(x)⊔μ(y)].
The definition in (53) states that a measure function μ is additive whenever the application of the function to the concatenation of two non-overlapping entities is identical to the sum of the application of the function to each entity. As Filip & Sutton (2017) put it, “an additive measure function tracks the part structure of the entities it measures” (Filip & Sutton 2017: 343). It follows from (53) that “if two objects have a weight of 2 and 3 kg respectively, then their concatenation has the weight of 5 kg” (Krifka 1989: 79). Similarly, a measure function like head-of is additive because the concatenation of two head of cattle and four head of cattle results in six head of cattle. Further examples of additive measure functions are pounds, meters, calories, etc. In contrast, there are also non-additive measure functions. Take degrees Celsius, for instance. If objects a and b have a temperature of 20 and 30 degrees Celsius, respectively, their concatenation doesn’t result in a temperature of 50 degrees Celsius.
Thus, Krifka treats counting as a special type of measuring, one whose units of measurement are concrete individuals or portions of a substance. In classifier languages, the additive measure function that provides concrete units is denoted by sortal classifiers, which must apply to cumulative nouns in order to derive countable constructions. As for mass/count languages, count nouns are lexically born with this counting function, while mass nouns lack them.
Krifka names the built-in counting function of count nouns nu, standing for ‘natural-unit’ (1989; 2003; 2007; 2008), or ou, standing for ‘object-unit’ (1995). It is modeled as an additive function μ that expresses a relation between quantities of P and a number n, as given in (54). nu measures P in terms of concrete units of counting.
- (54)
- nu(P⟨e,t⟩)(xe) = n
Mass nouns like blood are treated as one-place predicates, namely, as functions of type ⟨e,t⟩ that lack a built-in measure function, as illustrated in (55a). In contrast, count nouns like child are treated as two-place relations between numbers and entities, i.e., as functions of type ⟨n,⟨e,t⟩⟩, as shown in (55b).
- (55)
- a.
- ⟦Nblood⟧ = λx[blood(x)]
- b.
- ⟦Nchild⟧ = λnλx[child(x) ∧ nu(child)(x) = n]
Under this approach, numerals are arguments for nouns containing bult-in measure functions, saturating the n variable introduced by them. So, when (55b), a function from a number to a predicate, applies to a numeral like four, the result is a set of pluralities of children with cardinality 4.6
- (56)
- ⟦Nchild⟧(⟦four⟧) = (λnλx[child (x) ∧ nu(child)(x) = n])(4)
- = λx[child(x) ∧ nu(child)(x) = 4]
In languages that allow semantically plural bare nominals, like English, plural markers in bare nouns signal the existential closure of the number argument n, creating cumulative reference, as in (57).
- (57)
- ⟦Ndog-s⟧ = λx∃n[dog(x) ∧ nu(dog)(x) = n]
As for the selection of singular or plural forms of nouns when combined with numerals, it is enforced by syntactic agreement, since cardinals can only form a constituent with predicates containing an unsaturated number variable. So, while the plural -s in bare plurals in English has a semantics, its role in numeral+plural noun configurations is strictly syntactic.7
4.2 The semantics of Kaingang nouns
Based on the number neutrality and pervasive count meaning of Kaingang bare nouns, it can be tempting, at a first blush, to analyse individual and substance nouns in the language as cumulative atomic predicates, i.e., as exhibiting join-semilattice denotations with atoms at the bottom. In a mereology semantics, atoms can be defined absolutely, as given in (58), or relative to a predicate P, as defined in (59).
- (58)
- (Krifka 2007: 10)
- atom(x) ⟺ ¬∃y[y ⊏ x]
- (x is an atom iff it has no proper part.)
- (59)
- (Krifka 2007: 10)
- atom(P)(x) ⟺ P(x) ∧ ¬∃y[y ⊏ x ∧ P(y)]
- (x is an atom relative to a predicate P iff there isn’t a proper part of x of which P also holds.)
Given (58) and (59), an atomic predicate can be defined as in (60).
- (60)
- (Krifka 1989: 78)
- atomic(P) ⟺ ∀x[P(x) → ∃y[y ⊑ x ∧ atom(y)(P)]
- (A predicate P is atomic iff for every x that is P, there is a part of x that is an atom in P.)
Note that atomic(p) is true of both non-cumulative and cumulative atomic predicates. In other words, atomic(p) applies to nouns that denote sets of atoms, namely, (prototypical) singular nouns, as well as those that denote sets of atoms and their sums, i.e., plural nouns. As a result, on the assumption that atomicity is sufficient for nouns to be countable, an analysis of Kaingang nouns as cumulative atomic predicates would explain their count interpretation.
Nevertheless, it turns out that there is compelling crosslinguistic evidence indicating that atomicity is not the defining property of count nouns (Krifka 2007; Rothstein 2010; 2017). One piece of evidence comes from the so-called object mass nouns like furniture, luggage, kitchenware, jewellery, clothing, etc. Although these nouns have atoms in their denotations, and thereby can pattern in certain ways with count nouns – by allowing cardinal-based evaluations in comparative constructions and combining with count adjectives (Barner & Snedeker 2005) – this isn’t sufficient for them to be vested with the morphosyntax of count nouns. For example, in English and Brazilian Portuguese object mass nouns can neither inflect for number nor directly combine with numerals and other count quantity expressions.
Barner & Snedeker (2005) and Bale & Barner (2009), based on experimental tests, argue that the availability of cardinality-based readings of object mass nouns in quantity comparisons is evidence in support of an analysis of object mass nouns as semantically count, but morphosyntactically mass. The authors go further and claim that this class of nouns always triggers cardinality-based interpretations. But some studies have challenged this view (Rothstein 2016, 2017; Rothstein & Pires de Oliveira 2016). They demonstrate that object mass nouns can also be interpreted along other dimensions, such as weight and volume, and that this flexibility is context dependent. For instance, if (61a) is stated “in the context of choosing a moving truck, the volume of the furniture is relevant and not the number” (Rothstein 2017: 122). As such, (61a) can be true in a scenario where Bill has five pieces of furniture and John just three pieces, provided that the totality of John’s pieces of furniture occupy more space in a truck than Bill’s. Importantly, this is not the case with (61b), which is judged false in a scenario such as the one above. This indicates, observes Rothstein (2017), that pieces of furniture, as opposed to furniture, always forces a number-based reading.
- (61)
- a.
- John has more furniture than Bill, so he should use the larger moving truck.
- b.
- John has more pieces of furniture than Bill.
These cases hence reveal that the semantics of object mass nouns and singular count nouns differ. The cardinality-based evaluation of object mass nouns in comparisons seems to be favored by perceptual salience of the objects in their denotations. However, due to their mass meaning, they don’t impose any measuring dimension, leaving it for the context to arbitrate (Rothstein 2017). I believe Krifka’s theory (1989; 2007) offers a neat explanation of this flexible behavior of object mass nouns. They share atomicity with English prototypical count nouns and cumulativity with substance nouns. But, by lacking the quantized nature of count nouns, the cardinality dimension can’t be the only one available for them in comparison tasks (see Rothstein 2016 for a crosslinguistic perspective on object mass nouns).
A second piece of evidence, as Rothstein (2010; 2017) points out, involves count nouns such as rope, fence, wall, bouquet, table, etc. These nouns are non-atomic. Subparts of a rope can still be ropes, a fence or wall can be constituted by parts that are also fences or walls, and two bouquets or tables can be joined to form one bouquet or table. What counts as one fence or wall is context-sensitive, i.e., it can vary across utterance contexts. Without further specification, linking the count semantics of this class of nouns to atomic reference would be highly problematic, for it falls short of explaining their malleability regarding what constitutes one unit in their denotations.8
Accordingly, motivated by the crosslinguistic evidence above, I consider Krifka’s (2007) emphasis on the quantized/cumulative opposition better suited for explaining the semantics of the count/mass distinction than the atomic/non-atomic one. This is embedded in the machinery of his theory, which offers, I hope to demonstrate, an efficient toolbox for dealing with a language like Kaingang, where all nouns exhibit a count meaning.9
I thus propose to analyse the generalized counting strategy in Kaingang as a direct effect of the lexical meaning of nouns. Kaingang nouns are predicates that come from the lexicon already equipped with a context-sensitive built-in additive function à la Krifka (1989; 2007; 2008) which maps entities (individuals or portions) into numbers. Because all nouns have this quantizing function as part of their lexical meaning, they are already born quantized, which means that Kaingang root nouns are specified as being count. I assume that nouns give semantic information on the type of units in their denotations (Quine 1960). This is modeled as encoded in the counting functions of nouns. To indicate this information, I label the counting function of individual nouns iuC (individual-units), given in (62a), whereas the one of substance nouns is tagged puC (portion-units), given in (62b).
- (62)
- a.
- iuC(P⟨e,t⟩)(xe) = n [It measures P in context c in terms of individual-units.]
- b.
- puC(P⟨e,t⟩)(xe) = n [It measures P in context c in terms of portion-units.]
Taking gĩr ‘child’ and kyvénh ‘blood’ as illustrative nouns, the following lexical entries are assigned to Kaingang root nouns.10
- (63)
- a.
- ⟦
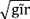 ⟧ = λx[child(x) ∧ iuC(child)(x) = n]
⟧ = λx[child(x) ∧ iuC(child)(x) = n]
- b.
- ⟦
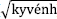 ⟧ = λx[blood(x) ∧ puC(blood)(x) = n]
⟧ = λx[blood(x) ∧ puC(blood)(x) = n]
Individual root nouns such as (63a) denote sets of individual-units, whereas substance root nouns like (63b) denote sets of portion-units. Their inherent counting function expresses a relation between quantities and a number, represented by the free variable n (see on the next page a detailed discussion of n as a free variable). The subscripted variable c in iu/pu encodes the context-dependence of what counts as one unit (Krifka 2007; Rothstein 2007; 2010). Under the analysis advanced here, the value of the variable c is conceived of as being pragmatically determined. The idea is that c provides the partition of the noun denotation by supplying the contextually salient individuals or portions to be accessed by the counting function iu/pu. The incorporation of c into the counting function allows us to represent the crucial role of utterance contexts in deriving the sets of portion-units in the denotation of substance nouns, which easily varies across contexts. For instance, if the sentence in (17), repeated here in (64), is uttered in context A, depicted in Figure 16, the set of units in the denotation of quantized goj will include the individuated portions (which are distinct in size and shape) in the gallon, in the glass, and forming the puddle, but exclude their proper parts. As a result, only the three (spatially) salient portions will be counted.
- (64)
The representation of puC as context-sensitive, hence, neatly captures the instability of substance count noun denotations, for portions are entities counted as contextual units. Moreover, by incorporating c into the built-in quantizing functions of count nouns, we show that it is the “context dependence of count nouns [that] explains the apparent non-quantization of nouns like fence, wall, hedge, bouquet” (Krifka 2007: 35), as well as of substance count nouns in Kaingang. As for count nouns that denote sets of stable individuals, like gĩr ‘child’ and fóg ‘non-indigenous person’, the sets of individuals will be constant for every value of c.11
Notice that in (63a–b) the number variable n is left free. I treat its value as contextually supplied, rather than saturated by a number argument. This departure from Krifka’s analysis not only allows cumulative and quantized predicates to be of the same type, i.e., ⟨e,t⟩, but it also prevents quantized nouns from gaining a cumulative denotation in an early stage of the derivation via existential closure of n at the n(p) or nump level, i.e., before they are converted into bare noun arguments.
This analysis shows that Kaingang bare nouns can be used to refer to pluralities selected from the denotation of quantized nouns, which indicates that plural interpretations of bare nouns uninflected for number don’t need cumulative reference at the n(p) or nump level.12
To implement my analysis, I represent Kaingang indefinite bare nouns in argument position as existentially bound choice functions.13 In prose, a choice function f maps a non-empty set onto one of its elements, which means that it’s a function of type ⟨⟨e,t⟩,e⟩. For instance, f applies to a quantized substance bare noun like kyvénh ‘blood’ and selects one of the portion-units that consist of blood. Let us take context A in (65) as an example. The salient drops of blood in this context have cardinality 3. Given that I treat contexts of utterance as supplying the value of the free variable n, in context A n turns out to be 3. That is, under this analysis, the n variable can be saturated by the cardinality of the portion-units that are contextually salient. As a result, the bare noun kyvénh in (65) denotes a quantized set with pluralities of cardinality 3 as elements, as given in (66a). Therefore, if, in context A, the choice function picks out the salient plurality of portions of blood with cardinality 3, e.g., the one that is encircled in (66a) and represented in (66b), then the sentence in (65) will be true.
- (65)
- Context A: You notice that some drops of blood [a total of 3 drops] fell from Fógtẽ’s nose.
- Kyvénh
- blood
- vỹ
- nom
- nãg ke
- drip
- ∅.
- pfv
- ‘Some (drops of) blood dripped.’
- (66)
- a.
- b.
In (67), I provide a compositional semantics of the truth conditions of the sentence in (65), abstracting away from tense.
- (67)
Note that the analysis implemented in (65–67) accounts for the plural interpretation of Kaingang bare nouns without associating it with a cumulative denotation. The contextual saturation of the n variable allowed us to demonstrate that in principle plural interpretations of bare nouns are not incompatible with assigning them a quantized denotation. In this regard, it is easy to see how the same analysis can be extended to contexts in which Kaingang bare nouns get a singular interpretation. In such scenarios the salient individual or portion is a singularity. As a consequence, the n variable will be saturated by 1. Thus, in the analysis illustrated in (65–67), by the time that a Kangang bare noun is type-shifted to an argument, it expresses a predicate which contains an unbound variable n, and hence is quantized relative to any contextual assignment of a value to n.
Alternatively, if we assume that the number-neutral meaning of Kaingang bare nouns necessarily relies on cumulative reference, this idea can be implemented by existentially binding the free variable n. This resource, as Krifka (1989) shows, gives rise to a cumulative interpretation. However, this is accomplished not at the root or np/nump level, but at a higher syntactic level after the quantized noun is inserted in the syntactic derivation. For concreteness, let us assume that this existential closure takes place at the level of the clause. By contrast, in languages that contain plural bare nouns, such as English, it’s the plural morpheme on the noun itself that introduces ∃-closure. Given that the number neutrality of Kaingang bare nouns isn’t associated with plural morphology, it can be assumed that the number variable n is unselectively bound by the existential operator that also binds the choice function variable f. Namely, the cumulative reading of Kangang bare nouns arises whenever the structure in (68) occurs at the clausal level (where ‘μC’ stands for the contextual measure function, either iuc or puc).
- (68)
- ∃f,n…[f(λx[P(x) ∧ μC(P)(x) = n]))]…
Thus, the approach proposed above envisages two possible ways of resolving the free variable n of Kaingang bare nouns, namely, contextual saturation or existential closure. Each of these mechanisms brings about its own semantic effects. The former always gives rise to a quantized bare noun with a contextually specified cardinality, while the latter leads to cumulative reference at the clause level (but not at the root level). Thus far both mechanisms are equally consistent with the Kaingang data. Only further research on the semantics of Kaingang bare nouns may provide supporting evidence to decide between the two strategies.
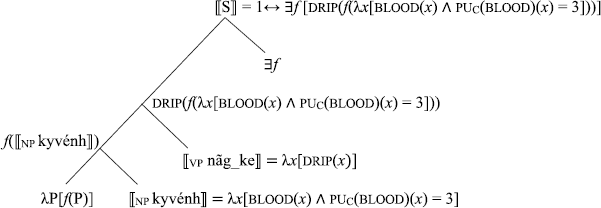
One immediate consequence of treating quantized nouns as predicates of type ⟨e,t⟩ is that numerals in Kaingang cannot denote arguments for the n variable, for whenever they combine with a noun n represents a free variable. To tackle this, I propose to model Kaingang numerals as predicate modifiers with a cardinality presupposition whose satisfaction depends upon the contextual value of the number variable n within quantized predicates. To illustrate this, (69) gives the lexical entry of the numeral tãgtũ ‘three’.
- (69)
- ⟦tãgtũ⟧ = λP⟨e,t⟩ λxe: ∀x[P(x) → iuC/puC(P)(x) = 3].P(x)
Under this theoretical solution, the cardinality presupposition targets the number within the measure function. It introduces the condition that the predicate modified by a numeral must display a certain contextual cardinality. The idea is that a numeral is only defined if its presupposition is satisfied by the contextually supplied number for the n variable of the noun it combines with. Given the lexical entry (69), hence, one-place quantized predicates become arguments of numerals. By functional application of the denotation of a numeral to a quantized predicate another quantized predicate is then created, but now with a cardinality presupposition passed on by the numeral. The schematic tree in (70) illustrates this procedure with the constituent kyvénh tãgtũ ‘three (portions of) blood’.
- (70)
In (70) the np denotation encodes the quantized nature of the noun, along with its ⟨e,t⟩ type, and as such it is ready to be the argument of the numeral, which, as a predicate modifier, i.e., an expression of type ⟨⟨e,t⟩,⟨e,t⟩⟩, applies to the NP and generates another predicate. At this stage, the presupposition of the numeral is projected into the derived predicate.14
The analysis outlined in (69) and (70) obviously does not intend to be an exhaustive account of numerals in the language. It was set forth in this paper, first and foremost, to show that it is in principle possible to provide a compositional treatment of a noun+numeral constituent in Kaingang when quantized nouns are represented as predicates of type ⟨e,t⟩ containing in their internal structure a counting function modeled along the lines of Krifka.
To summarize, the analysis advanced here treats all nouns in Kaingang as lexically count. Nouns express quantized predicates of type ⟨e,t⟩ containing a built-in counting function that measures quantities in terms of individual- or portion-units. The context-dependence of what counts as a unit is incorporated into the function via a contextual variable c, as in Krifka (2007). This guarantees that the units are evaluated relative to the utterance context. Further, the number variable of the counting function is left free, allowing its value to be contextually supplied and ensuring that the root noun is quantized, rather than cumulative (unlike English plural count nouns). This solution entails that individual and substance nouns in Kaingang can still be quantized at the point at which they are converted into bare noun arguments with an indefinite interpretation. This is so even when bare nouns denote pluralities. By representing indefinite bare nouns as introducing a silent choice function, I showed that pluralities can be selected from the extension of quantized nouns whenever their contextual cardinality is > 1. Finally, I also suggested that the number-neutral meaning of bare nouns can be analysed as arising at the clause level from the option of unselectively binding the number variable via the existential operator that also binds the choice function variable.
5 Extending the analysis to Yudja
In this section, I shall first briefly discuss Lima’s (2014a; b) analysis of Yudja nouns. By doing so I aim to highlight what I consider to be the explanatory advantage of an approach that treats the count nature of nouns in Yudja as resulting from a quantized denotation.
In Yudja nouns are number neutral, can be bare in all argument positions, and allow (in)definite or kind readings. Numerals can combine directly with individual and substance nouns. Substance nouns are modified by numerals even when used to refer to non-conventionalized portion-units, as in (71).
- (71)
- Txabïu
- three
- apeta
- blood
- pe∼pe∼pe. (Yudja)
- drip∼red
- ‘Three drops of blood dripped.’
Quantity words also select for substance nouns, in most cases triggering a cardinal interpretation. In (72) itxïbï ‘many’ exhibits the cardinal reading of a large number of contextually supplied portion-units of water.
- (72)
- Itxïbï
- many
- y’a
- water
- a’i. (Yudja)
- here
- ‘There are many (portions of) water here.’
Quantity judgement experiments show that Yudja speakers strongly favor a number-based evaluation of substance nouns in comparative constructions (Lima 2014a; b). In scenarios, for instance, containing a collection of one or two portions of flour side-by-side with another collection of multiple portions of the same substance, the majority of Yudja speakers responded to the stimuli sentences (73) and (74) – which contain the quantity expressions bitu ‘more’ and itxïbï ‘many’, respectively – by choosing the collection with a greater number of portion-units of flour, regardless of their total volume.
- (73)
- Ma de
- who
- bitu
- more
- asa
- flour
- dju a’au? (Yudja)
- have
- ‘Who has more flour?’
- (74)
- Ma de
- who
- itxïbï
- many
- asa
- flour
- dju a’au? (Yudja)
- have
- ‘Who has many portions of flour?’
Based on constructions such as (71–74) and their predominant cardinality-based readings, which parallel the Kaingang data I have reported in this paper, Lima (2014a; b) claims that all nouns in Yudja are count. However, she offers an analysis that differs from mine. She proposes a semantics for Yudja nouns in which root nouns denote kinds, as illustrated in the lexical entry of asa ‘flour’ in (75), where flour in capital letters is a standard notation for kinds.
- (75)
- (Adapted from Lima 2014a: 100)
- ⟦
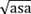 ⟧ = flour
⟧ = flour
As for the predicate meaning of nouns, Lima (2014a; b) proposes that it is morphologically derived by combining the root with a silent operator, called ko (kind-to-object), which maps a kind “to a property that is true of atomic individuals and their sums” (Lima 2014a: 100). More precisely, it applies to a kind k and returns “a number neutral property of atomic individuals and their sums who are members of k” (Lima 2014b: 537), as in (76a–b).15
- (76)
- (Adapted from Lima 2014a: 100)
- a.
- ko = λk: k ∊ K. λx.at*(x)(k)
- b.
-
ko(⟦
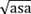 ⟧) = λx.at*(x)(flour)
⟧) = λx.at*(x)(flour)
Note that (76b) is the derived denotation of asa, i.e., a set of atomic and sum realizations of the kind flour. Although Lima doesn’t represent the context in (76a–b), she claims that the atoms of substance nouns are spatially specified.16 By having an atomic join-semilattice denotation, (76b) is cumulative, i.e., a set which is closed under sum formation, as already observed by Deal (2017). Consequently, (76b) is not a quantized predicate, although it contains (contextually determined) atomic parts. This reveals that in Lima’s approach the property ensuring that all nouns are count is atomicity, and not quantization, as on my account.
However, although Lima’s analysis can apply specifically to Yudja, it falls short of allowing for generalization to other languages. As discussed in section 4, there is compelling crosslinguistic evidence that denoting a set of atoms and their sums, i.e., having a cumulative atomic reference, doesn’t guarantee that a noun is count. This is attested by object mass nouns (like furniture) in mass/count languages, which denote an atomic join-semilattice structure. Further evidence that a sum denotation with atoms as parts doesn’t ensure that a noun is lexically count can also come from classifier languages. For instance, Doetjes (1997) and Cheng, Doetjes & Sybesma (2008) propose that Mandarin encodes a parts-based distinction, rather than a sums-based one. Roughly speaking, all Mandarin nouns are cumulative, and their main division is between atomic and non-atomic join-semilattice denotations. If such an analysis is on the right track, it can be interpreted as showing that Mandarin distinguishes atomic from non-atomic mass nouns, but not count nouns from mass nouns.
Indeed, if across languages being a count noun means being quantized, and if numerals (and other count quantity expressions) only select for quantized constructions, to maintain the semantics in (75) and (76a–b) we then would have to complement it with one of two options. We could stipulate a covert counting function that applies to Yudja substance nouns and turns them into quantized predicates, which then can be complements of numerals, as Deal (2017) proposes. Or we could treat the counting function as part of the lexical meaning of Yudja numerals (and other count quantity expressions), along the lines of Wilhelm’s (2008) analysis of Dëne Sųłiné, an Athabascan language. However, either option would undermine Lima’s claim that all Yudja nouns are count.
Rather, I believe that my account of Kaingang nouns as inherently quantized predicates of type ⟨e,t⟩ can be extended to Yudja nouns. In this way, I propose to eliminate the operator ko and replace the lexical entry of a (substance) noun like asa in (75) by (77), analogous to my analysis of Kaingang.
- (77)
- ⟦
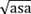 ⟧ = λx[flour(x) ∧ puC(flour)(x) = n]
⟧ = λx[flour(x) ∧ puC(flour)(x) = n]
In (77) asa is a root noun of type ⟨e,t⟩ with a context-sensitive counting function as part of its lexical meaning. puC maps a quantity of flour onto a number n of contextually specified portion-units of flour. Thus, just like Kaingang nouns, the count nature of Yudja nouns arises from their quantized semantics.
Concerning the number neutrality of Yudja bare nouns, like in Kaingang, it does not need to be associated with a lexical cumulative denotation, as shown in section 4. In (77) the number variable is left free, which means that it can be contextually resolved or bound by ∃-closure. The latter strategy gives rise to cumulative reference at the phrasal level, not for the root noun. That is, a cumulative denotation can perfectly well result from a semantic operation applying at a higher syntactic level after the root noun is inserted in the syntactic derivation.17
As a hypothetical exercise, I posit that the kind interpretation of Yudja bare nouns is derived via a type-shifting operation triggered by a type mismatch between them and kind-level predicates, which require a kind argument. It can be modeled as a three-step mechanism. First, the n variable is existentially closed at the np layer. That is, the quantized denotation of the root noun is projected into the np layer, where the n variable is then targeted by ∃. Secondly, the ∃-binder above the root is demoted to a position after the x argument.18 The resulting predicate then has a cumulative reference. Finally, Chierchia’s (1998a) kind-shifting operator ⋂ applies to this predicate and maps it onto a kind, as illustrated below.
- (78)
- a.
- ⟦
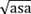 ⟧ = λx[flour(x) ∧ puC(flour)(x) = n]
⟧ = λx[flour(x) ∧ puC(flour)(x) = n]
- b.
- [∃]⟦
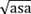 ⟧ = λx∃n[flour(x) ∧ puC(flour)(x) = n] by ∃-closure and demoting of ∃
⟧ = λx∃n[flour(x) ∧ puC(flour)(x) = n] by ∃-closure and demoting of ∃
- c.
- ⋂λx∃n[flour(x) ∧ puC(flour)(x) = n] = flour by the kind-shifting operator ⋂
Note that in (78b) I allow Yudja to differ from Kaingang with respect to how the existential closure of the n variable occurs. In (78b) ∃ must only bind n, as opposed to Kaingang, in which ∃ unselectively binds a choice function variable and n. Such differences in the way that ∃-closure is implemented are not a problem for the analysis. How the existential binding of n is accomplished may very well vary across languages. Crucially, in (78a–c) I adopt the approach in Krifka (2003), where bare nouns are predicates (or properties in their intensional version) and can be shifted to kinds in appropriate contexts via the ⋂ operator, as opposed to Krifka (1995), where nouns start out as kind-denoting expressions.19
In the next section, I deal with a potential challenge to the claim that all nouns in Yudja and Kaingang are count.
6 A Challenge for the Analysis
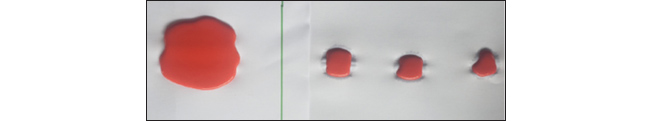
Data from a version of the quantity judgement tasks may at a first glance pose a challenge to the thesis that all nouns in Kaingang and Yudja are inherently count. It comes from scenarios exhibiting pictures of two portions of the same substance, differing in volume, as shown in Figures 17 and 18.
When shown the single-unit situations above followed by the target sentence (79), two out of the three Kaingang consultants said that it wouldn’t make sense to ask it, given that the units are numerically identical, whereas one speaker pointed to the unit with the larger volume.20
- (79)
- Ũ
- indef
- nỹ
- q
- tỹ
- sbj
- kyvénh/kakanẽ
- blood/fruit
- mẽ
- more
- nĩ?
- asp
- ‘Who has more blood/fruit?’
The immediate stance is to interpret the larger unit answer as a case of assessment along the volume dimension. On the assumption that a volume interpretation is associated with a mass denotation, one then can argue that there are constructions in Kaingang and Yudja where substance nouns are not quantized and as such cannot be inherently count, so that their count interpretation must involve a silent unitizing operator. As a result, although languages like Kaingang and Yudja morphosyntactically don’t encode the mass/count distinction, the volume evaluation attested in quantity judgments of single-unit scenarios would suggest that a semantic distinction is available. This is basically Deal’s (2017) reanalysis of Yudja nouns as sharing the semantics she assigns to Nez Perce, a Sahaptian language. That is, Yudja individual nouns are lexically quantized, while substance nouns are born with a mass denotation, which then is mapped onto a quantized one via Deal’s (2017) silent atomization operator.21
I don’t think that the availability of a volume-like interpretation can immediately constitute conclusive evidence against lexically quantized substance nouns. Instead, it can be accommodated by a coercion-based mechanism that licenses a mass interpretation of count nouns which maps individuals onto their proper parts, along the lines of Lewis’s Universal Grinder operation (Pelletier 1975; Cheng, Doetjes & Sybesma 2008; Rothstein 2017). This operation was introduced to account for constructions like (80a–b), where the count nouns dog and car are coerced to denote the stuff individuals are made up of. Strictly speaking, it’s in principle possible to offer an analysis of the Yudja and Kaingang data that posits a count-to-mass interpretation shift triggered by (comparison) scenarios that coerce nouns into a mass reading.
- (80)
- a.
- After the accident there was dog all over the highway.
- b.
- (Moravcsik 2017: 440)
- Too many people are driving too much car these days.
However, I will not follow this path here. Rather, in a more hypothetical fashion, I will tackle the apparent problem raised by single-portion situations. I propose to reinterpret them in a manner that makes the option for the larger unit compatible with a cardinality judgement.
For exposition, let us focus on a substance noun like kyvénh ‘blood’. The idea is that Kaingang speakers can conclude that the single unit on the left side of the picture is larger than the one on the right side by drawing an interpretation that uses the smaller portion as a parameter-unit of comparison on the basis of which they partition the larger portion. This is achieved by reasoning that the larger portion can be portioned into two or more units, one of which, at least, is of the same size as the one displayed by the smaller portion. I claim that this partition reading is a last resort interpretation of comparisons triggered by single-portion scenarios. Strictly speaking, Kaingang speakers resort to it to enforce a cardinality reading in these scenarios.
In set theory talk, a partition of a set can be defined as in (81).
- (81)
- (Adapted from Schwarzschild 1996: 64)
- Y is a partition of a set A iff:
- i.
- Y is a set of subsets of A.
- ii.
- Every member of A belongs to a set in Y.
- iii.
- ∅ does not belong to Y.
- iv.
- No two members of Y overlap.
- v.
- ∪Y = A
I propose to extend the notion of a partition above to proper parts of an individual or portion by reinstating (81) as (83). (82) is the definition of proper parthood.
- (82)
- ∀x,y[x ⊏ y ⟺ x ⊑ y ∧ ¬(x = y)]
- (x is a proper part of y iff x is part of y and x is distinct from y.)
- (83)
- Y is a partition of an individual/portion-unit x – part(Y)(x) – iff:
- i.
- Y is a set of proper parts of x.
- ii.
- Every proper part of x belongs to Y.
- iii.
- No two members of Y overlap.
- iv.
- ⊔Y = x
Thus, the partition-based reasoning that makes available a numerosity interpretation of (84), given the context depicted in Figure 17, is formalized in (85). Crucially, (85) doesn’t represent the truth conditions of (84). (85) is just a formal representation, cast in a long formula, of an interpretation derived via a pragmatic reasoning that underlies the cardinality judgement of (84) in single-unit contexts.
- (84)
- Pakój
- Pakój
- vỹ
- NOM
- kyvénh
- blood
- mẽ
- more
- nĩ
- ASP
- Maria
- Maria
- ve kỹ.
- than
- ‘Pakój has more blood than Maria.’
- (85)
- ∃x∃y∃Y∃z1…∃zn[blood-of-pakój(x) ∧ puC(blood-of-pakój)(x) = 1 ∧ blood-of-maria(y) ∧ puC(blood-of-maria)(y) = 1 ∧ part(Y)(x) ∧ (z1 ∊ Y) ∧ (zn ∊ Y) ∧ ¬(z1 = zn) ∧ (same-size(z1, y) ∨…∨ same-size(zn, y))]
Notice that in (85) the minimal cardinality requirement on the partition is two, as indicated by the existentially bound variables z1 and zn and the negation of identity, expressed by ¬(z1 = zn). This allows flexibility in the ways a unit can be partitioned, the cardinality of the partition, and the sizes of its cells, so long as the partition contains at least one unit-cell with the same size of the unit that serves as the parameter of comparison. This last condition is ensured by the disjunction(s) of the two-place predicate same-size. Importantly, (85) does not involve evaluating the overall size of the partition. Sameness of size between one unit of the partition and the parameter-unit is sufficient for opting for the larger portion.
I propose that the speaker arrives at the interpretation in (85) via the following pragmatic steps involving Grice’s cooperative principle (Grice 1975).22
- (86)
- a.
- The interlocutor asks a question that implies there should be a cardinality difference between two quantities of context-dependent portion-units of blood.
- b.
- However, the most contextually salient portion-units result in a cardinality of 1 for both quantities.
- c.
- The consultant wants to be cooperative.
- d.
- The consultant adjusts the value of the contextual variable c to one that leads to a cardinality difference between the two quantities, e.g., by partitioning the larger portion-unit of blood.
As for the comparison involving the individual-denoting noun kakanẽ ‘fruit’, it is easy to see how the same rationale in (86) can also be carried over to this case to give rise to an interpretation like (85). Importantly, there were no volume-like comparisons, in single-unit scenarios, with nouns denoting animate beings and artefacts, such as gĩr ‘child’ and nĩgja ‘chair, bench’, respectively. The possibility of a partition interpretation like in (85) of Kaingang substance nouns and a subset of its individual nouns in single-unit contexts can be attributed to language-independent factors involving world perception/knowledge. Because the ‘minimal’ parts of a blood-unit or fruit-unit that are still considered blood or fruits can go down to quite small parts (particularly in the case of blood), contextually larger units in the extension of kyvénh ‘blood’ or kakanẽ ‘fruit’ become privileged candidates to be portioned in scenarios where the cardinality of the quantities under comparison is 1.
Thus, by suggesting the alternative interpretation modeled in (85), which relies on the notion of a partition set imposed on the larger portion of a single-unit scenario, I think I have shown that the assessment of one of the speakers isn’t in principle incompatible with a cardinality-based comparison. (85), as well as its version with the noun kakanẽ ‘fruit’, rescue nouns from a mass-like interpretation. In short, partitions of the larger individuals or portions of single-unit situations can be portrayed as a strategy at the disposal of Kaingang speakers to make available, whenever it is relevant for communicative purposes, an alternative cardinal assessment that circumvents the numerical identity of these scenarios.
To conclude, it is worth stressing one point already mentioned in this subsection, which is independent of whether the analysis offered here is correct. The fact that all nouns are lexically count in Kaingang and Yudja doesn’t entail that in these languages the denotation of nouns cannot be measured along other dimensions, such as volume and size. What it implies is that, given the quantized basic denotation of all nouns, the eventual availability of any mass-like interpretations must be derived via a (covert) massifying operation. In this regard, languages like Kaingang and Yudja would sit at the extreme opposite end of classifier languages on the spectrum of nominal countability. The latter requires classifiers to turn cumulative nouns into quantized predicates, while the former would need a (silent) massifying function to create a mass interpretation out of quantized nouns.
7 Crosslinguistic Contributions
The growing body of work on nominal countability across understudied languages in the last fifteen years shows that there is a significant variation in the ways that languages encode the mass/count distinction in their nominal domain (Deal 2017; Davis 2014; Gillon 2010; Lima 2014a; b; Lima & Rothstein 2020; Mathieu 2012; Wilhelm 2008; Wiltschko 2012; among others). Some of these studies, as pointed out by Lima & Rothstein (2020), have challenged two core assumptions in earlier typological approaches, such as in Greenberg (1974) and Chierchia (1998a; b).
First, the assumption that in mass/count languages mass nouns cannot be directly modified by numerals, unless they undergo conventionalized packaging or receive a taxonomic reading, doesn’t always hold. Deal (2017) demonstrates that this is not the case for Nez Perce. All Nez Perce nouns combine with numerals without the intervention of classifiers. The morpho-syntactic realization of the mass/count distinction shows up in the plural marking on adjectives when they interact with quantifiers. In a constituent like [Q[Adj+noun]] adjectives must be pluralized when combined with individual nouns, but not with substance nouns.
A second challenged assumption is the idea that in mass/count languages where nouns inflect for number count nouns can be pluralized, while mass nouns cannot. There is an attested number of languages that pluralize (substance) mass nouns. For instance, Innu-aimun (Gillon 2010) Ojibwe (Mathieu 2012), both Algonquian languages, as well as St’át’imcets (Davis 2014), a Salish language, allow mass nouns to inflect for number. Recent studies, all carried out as part of a typological research project on countability in Brazilian Indigenous languages coordinated by Suzi Lima and Susan Rothstein, have also shown that some of the languages under investigation that encode the mass/count distinction can pluralize (substance) mass nouns. Such languages are Tenetehára (Chamorro & Duarte 2020) and Sakurabiat (Galucio & Costa 2020), both Tupi languages, Terena (Sanchez-Mendes et al. 2020), an Arawakan language, as well as Taurepang (Costa 2020) and Ye′kwana (Costa 2020), both Cariban languages. Importantly, in Sakurabiat, Taurepang, and Ye′kwana the pluralization of substance nouns gives rise to a plurality of portions reading, rather than an abundance one.23
Kaingang provides some important contributions to the understanding of nominal countability across languages. One relevant contribution is that Kaingang joins Yudja (Lima 2014a; b) as evidence that the mass/count distinction isn’t a language universal in the nominal domain. This is so because the data assembled in section 3 show that Kaingang, like Yudja, lacks any morphosyntactic or semantic properties associated with the mass/count distinction. Such crosslinguistic commonalities indicate that the opposition between mass and count nouns plays no role in these languages.24
Another contribution of Kaingang relates to mismatches between the linguistic encoding of countability and the conceptual or perceptual distinction between stuff and objects. As discussed in section 4, many count/mass languages contain object mass nouns like furniture, luggage, etc., and a single language can even exhibit mass/count doublets, like in English the pairs footwear/shoes, mail/letters, change/coins, etc. These cases of asymmetries in the mapping from the stuff/object opposition to the mass/count distinction tell us that atomicity isn’t a sufficient condition for a noun to be count (Krifka 2007; Rothstein 2010; 2017).
Indeed, if my analysis is correct, Kaingang and Yudja reveal that the asymmetry is stronger, since even substance nouns can be lexically count. This means that, together with fence-type nouns, i.e., those whose individuals in their denotations are contextually determined, like bouquet and wall, as already discussed by Rothstein (2017), the existence of count substance nouns proves that atomicity isn’t even a necessary condition for a noun to be count.
Let me end this section with the typological picture that emerges from the Krifka-inspired generalizations suggested in this paper. Namely, (i) the claim that the obligatory count meaning of Kaingang and Yudja nouns, associated with the crosslinguistic facts above, constitute evidence that the defining property of grammatical counting is quantization; and (ii) the view that the count/mass opposition is a semantic phenomenon derived from the quantized/cumulative distinction. Placed on a scale of nominal countability, classifier languages like Mandarin fall on one extreme as languages where all nouns are inherently cumulative. This type of languages encodes a parts-based distinction, i.e., they distinguish atomic from non-atomic mass nouns. In these languages the count interpretation is associated with classifiers, which apply to nouns and map their cumulative denotation onto quantized ones. On the other extreme of the scale is the type of languages where all nouns are lexically quantized, such as Yudja and Kaingang. In these languages cumulative denotation is derived via existential closure of their number variable. Somewhere between these two poles reside the so-called mass/count languages, such as English and Brazilian Portuguese. These languages lexically encode the quantized/cumulative distinction, i.e., they differentiate cumulative nouns from quantized ones.
8 Conclusion
In this paper I have demonstrated that individual and substance nouns in Kaingang are interpreted as count nouns. Both types of nouns pattern alike regarding the grammar of counting, i.e., there are no morphosyntactic or semantic features that distinguish them in this domain. Based on these findings, I have argued that all nouns in Kaingang are lexically count. I have modeled this common feature of all nouns by proposing that they express quantized predicates of type ⟨e,t⟩ equipped with a context-sensitive built-in counting function that measures quantities in terms of individual- or portion-units. Further, in consonance with Krifka (2007), I have argued for the crosslinguistic explanatory advantage of the quantization property over atomicity as the defining property of count nouns. Finally, if my analysis of Kaingang nouns is correct, it has provided additional crosslinguistic evidence that challenges the view that the mass/count distinction, at least in the nominal domain, is a language universal. To conclude, it should be mentioned that a path for future investigation will be to test the main claims of this paper against a broader sample of Kaingang speakers in an experimental psycholinguistic study.
Abbreviations
1sg = first person singular; 1pl = first person plural; 1poss.pl = first person possessive plural; 3sg.f = third person singular (feminine); 3pl.f = third person plural (feminine); asp = aspect; fut = future; indef = indefinite; neg = negation; nom = nominative; pfv = perfective; q = question particle; recp = reciprocal; red = reduplication; sbj = subject; top = topic.
Notes
- Following Nascimento (1995), I assume a zero perfective marker (Ø) in clauses which are not overtly marked for viewpoint aspect. [^]
- I’m indebted to Lima for suggesting this test to me. [^]
- The noun kur is ambiguous, it means clothes/clothing or fabric. This fact justifies the occurrence of kur in both lists. [^]
- Like in English, in Yudja container phrases can also have a measuring interpretation. It is still unclear whether a measuring interpretation of Kaingang container phrases is also available. For an in-depth discussion of container phrases in Yudja and all their available interpretations, see Lima (2014a; 2016). [^]
- Another property often assigned to substance mass nouns is divisiveness, which is the opposite of quantization. A predicate P is divisive (at least down to a certain threshold) if, and only if, every proper part of something that is P is also P. Quantized predicates are, therefore, neither cumulative nor divisive. Given that all nouns in Kaingang are count, it naturally falls out from this that divisiveness doesn’t play any crucial role in the noun inventory of the language. [^]
- Krifka (1995) also proposes an alternative analysis, according to which in non-classifier languages the measure function nu/ou is part of the meaning of numerals, whereas in classifier languages numerals combine first with classifiers. In my analysis of Kaingang nouns, I adopt the view in Krifka (1989; 2007), i.e., that count nouns incorporate a measure function in their semantics. For defense of the theory that classifiers and silent measure functions combine with numerals, see Wilhelm (2008) and Bale & Coon (2014). [^]
- Evidence for plural inflection as agreement comes from plural agreement with decimal fractions in English and languages where numerals greater than one obligatorily combine with singular nouns, like Turkish and Hungarian (Ionin & Matushansky 2006; Krifka 2007). [^]
- To account for fence-type nouns, Rothstein (2010; 2017) proposes a distinction between ‘natural atomicity’ and ‘semantic atomicity’. Natural atoms are inherently discrete entities, while semantic atoms are defined as atoms relative to contexts. In her analysis all count nouns denote sets of semantic atoms. The atoms associated with prototypical count nouns usually coincide with natural atoms, while those in the denotation of fence-type count nouns, due to their context sensitivity, do not. [^]
- For an alternative treatment of the mass/count distinction that also doesn’t rely on the notion of atomicity, see De Vries & Tsoulas (2023). Their analysis is inspired by Landman’s (2020) iceberg semantics and employs the notions of overlap versus disjointness to account for the distinction. [^]
- The term ‘root nouns’ refers to the members of the open-class vocabulary categorized as nouns. The use of the symbol √ to represent roots is a standard notation in Distributive Morphology (Marantz 1997; 2001). [^]
- A note of caution is in order here. I’m careful not to call individuals and portions atoms because they have parthood structures and as such clearly don’t fall under (58), namely, the absolute definition of atoms. I can’t see how atom(x) could be true of the entities of our daily experience. However, once the semantics of fence-type and substance count nouns is linked to context sensitivity in the way proposed above, it follows from this that the contextual units of quantized predicates coincide with Rothstein’s contextual atoms, i.e., atoms relative to contexts (see footnote 8). As a result, atom(p)(x), as defined in (59), is true of the entities that are (contextual) units in the denotation of Kaingang nouns. Note that this doesn’t contradict the claim that “the essential feature [of count nouns] is quantization, not atomicity” (Krifka 2007:28). Although quant(p) entails atomic(p), as defined in (60), the reverse direction of the entailment doesn’t hold. [^]
- This doesn’t reveal that the free variable solution is empirically superior to Krifka’s approach. It is just an alternative to the latter which shows that we can account for plural readings of Kaingang bare nouns without requiring that a quantized noun be first converted into a cumulative one. [^]
- This representation of indefinite bare nouns as choice functions isn’t a mere posit to oil the wheels of the analysis above. The choice function theory of indefinites, introduced by Reinhart (1997) and Winter (1997), was specially designed to account for the exceptional scope behavior of indefinites with respect to syntactic islands such as if-clauses. The main advantage of a choice function analysis is that it explains the scope interaction of indefinites with respect to other operators without resorting to LF movement. The existential closure of choice functions at any level captures their interpretations. Given that Kaingang indefinite bare nouns scopally interact with other operators such as negation, intensional verbs, and if-clauses, as well as exhibit intermediate scope readings, treating them as contributing an existentially closed choice function is an empirically motivated solution. To illustrate this, the indefinite bare noun kanhgág ‘Kaingang’, as we see in (i), can be interpreted outside or within the scope of the if-clause.
This is a topic far beyond the reach of the present paper. However, this brief note was necessary to elucidate the timely use of choice functions above. [^]
- (i)
- ✔Context A: There will be many Kaingangs at the party. You, the party singer, are convinced that if a particular Kaingang (named Fógtẽ) arrives there, you will sing the song Garçom.
- ✔Context B: If any Kaingang arrives at the party, whoever they are, you will sing the song Garçom.
- Fénhta
- party
- ki
- at
- kanhgág
- Kaingang
- jun
- arrive
- mũra,
- if,
- sóg
- 1sg
- Garçom
- Garçom
- jãn
- sing
- ke
- fut
- mũ.
- asp
- ‘If a Kaingang arrives at the party, I will sing Garçom.’
- One anonymous reviewer raised the issue that the account above entails the presupposition failure of numerals in out-of-the-blue statements. I don’t think this is a real problem for the analysis, because of the pervasiveness of presupposition accommodation. The presupposition can be accommodated by leaving it up to the hearer to imagine a context compatible with the presupposition. [^]
- Lima analysis of Yudja nouns is formulated in an intensional language, where a world variable is part of the lexical entries. KO maps a kind into a property, i.e., into the set of atoms and their sums in a world of evaluation w. KO contains a function AT* “that maps an individual x, a world w and a kind k to the truth value 1 if and only if x is the sum of atomic parts of k(w)” (Lima 2014b: 537). For expository purposes, (75) and (76a–b) are simplified extensional versions of the original denotations. [^]
- To model portions as atoms, Lima (2014a; b) relies on mereotopological notions (Casati & Varzi 1999) such as (self)-connectedness and maximal self-connected portions. Drops of blood, portions of sand, etc., are represented as atoms whose individuations are spatially specified. Given that the focus of the present section is mainly the semantics of (76b), it is not essential here how Lima (2014a; b) independently models portions as atoms. [^]
- Note that the analysis of Yudja nouns in (77) forces us to treat Yudja numerals like the way we analysed Kaingang numerals. However, this point is not essential here. The lexical entry for nouns like asa could denote a relation between numbers and predicates, as in Krifka (1989; 2007). What is crucial here is to treat Yudja nouns as having built-in quantizing functions. Either account can accomplish this requirement. [^]
- For previous use of the demoting mechanism in the literature, see Chung & Ladusaw (2003). [^]
- It is still unclear whether Kaingang bare nouns can also be used to refer to kinds. [^]
- Yudja speakers (Lima 2014a) favored the volume interpretation of substance nouns in single-unit comparisons. [^]
- Doetjes (2021) gives an alternative explanation of the Yudja data. She argues that the availability of volume readings in single-unit situations can be an effect of the quantity word bitu ‘more’, rather than the semantics of the noun. Volume readings would demonstrate that the quantity expression bitu, like its English counterpart more, doesn’t impose a comparison in terms of number, i.e., that it would allow interpretations along other quantity measures, like volume, whenever the context favors them. [^]
- I am indebted here to one of the reviewers for suggesting the pragmatic reasoning in (86). [^]
- Rothstein (2021) analyses plural substance nouns in Sakurabiat, Taurepang, and Ye′kwana as denoting a set of contextually specified discrete portions closed under sum. Importantly, these nouns are not countable, despite their portion readings. This fact provides further crosslinguistic evidence that having discrete entities in their denotations isn’t a sufficient condition for nouns to be countable. In order to be so, they have to be quantized. [^]
- Halkomelem, a Salish language, may also challenge the universality of the count/mass distinction (Wiltschko 2012) However, I shall mention here that, to my knowledge, the quantity judgement test hasn’t been applied to Halkomelem. However, with respect to most of the other tests in section 3, Halkomelem (Wiltschko 2012) seems to behave like Yudja and Kaingang. For a view that casts doubt on Wiltschko’s analysis, see Davis (2014). [^]
Acknowledgements
I would like to express my thanks to the Kaingang consultants who generously provided the data: Darci Fógtẽ Bernardo, Cristielly Pakój Bandeira, and Danusa Kórig Bernardo Fernandes. I would also like to thank Amy Rose Deal, Lisa Matthewson, Hotze Rullmann, Marcin Morzycki, Roberta Pires de Oliveira, Suzi Lima, the UBC Semantics Reading Group, and three anonymous reviewers for their valuable comments and suggestions. All errors are my responsibility.
Competing interests
The author has no competing interests to declare.
References
Bale, Alan & Barner, David. 2009. The interpretation of functional heads: using comparatives to explore the mass/count distinction. Journal of Semantics 26(3). 217–52. DOI: http://doi.org/10.1093/jos/ffp003
Bale, Alan & Coon, Jessica. 2014. Classifiers are for numerals, not for nouns: Consequences for the mass/count distinction. Linguistic Inquiry 45. 695–707. DOI: http://doi.org/10.1162/LING_a_00170
Barner, David & Snedeker, Jesse. 2005. Quantity judgments and individuation: Evidence that mass nouns count. Cognition 97(1). 41–66. DOI: http://doi.org/10.1016/j.cognition.2004.06.009
Bloomfield, Leonard. 1933. Language. New York: Holt, Rinehart & Winston.
Bochnak, M. Ryan & Matthewson, Lisa. 2020. Techniques in complex semantic fieldwork. Annual Review of Linguistics 6. 261–283. DOI: http://doi.org/10.1146/annurev-linguistics-011619-030452
Bohnemeyer, Jürgen. 2015. A practical epistemology for semantic elicitation in the field and elsewhere. In Bochnak, Ryan & Matthewson, Lisa (eds.), Methodologies in semantic fieldwork, 13–46. Oxford: Oxford University Press. DOI: http://doi.org/10.1093/acprof:oso/9780190212339.003.0002
Casati, Robert & Varzi, Achille C. 1999. Parts and places: The structures of spatial representation. Cambridge, MA: MIT Press.
Chamorro, Pilar & Duarte, Fábio Bonfim. 2020. On the semantic properties of mass and count nouns in Guajajára (Tenetehára). Linguistic Variation 20(2). 366–381. DOI: http://doi.org/10.1075/lv.00027.cha
Cheng, Lisa L. S. & Doetjes, Jenny & Sybesma, Rint. 2008. How universal is the universal grinder? In van Koppen, Marjo & Botma, Bert (eds.), Linguistics in the Netherlands 2008, 50–62. Amsterdam: John Benjamins. DOI: http://doi.org/10.1075/avt.25.08che
Chierchia, Gennaro. 1998a. Plurality of mass nouns and the notion of ‘semantic parameter’. Events and grammar, 53–103. Dordrecht: Kluwer. DOI: http://doi.org/10.1007/978-94-011-3969-4_4
Chierchia, Gennaro. 1998b. Reference to kinds across languages. Natural Language Semantics 6. 339–405. DOI: http://doi.org/10.1023/A:1008324218506
Chung, Sandra & Ladusaw, William A. 2003. Restriction and saturation. Cambridge, MA: MIT Press. DOI: http://doi.org/10.7551/mitpress/5927.001.0001
Costa, Isabella Coutinho. 2020. The count/mass distinction in Taurepang. Linguistic Variation 20(2). 352–365. DOI: http://doi.org/10.1075/lv.00026.cou
Costa, Isabella Coutinho. 2020. The count/mass distinction in Ye′kwana. Linguistic Variation 20(2). 409–419. DOI: http://doi.org/10.1075/lv.00030.cou
Davis, Henry. 2014. The count-mass distinction in St’át’imcets (and beyond). Papers for the Forty-ninth International Conference on Salish and Neighboring Languages (ICSNL 49), University of British Columbia Working Papers in Linguistics 37. 155–181.
Deal, Amy Rose. 2017. Countability distinctions and semantic variation. Natural Language Semantics 25(2). 125–171. DOI: http://doi.org/10.1007/s11050-017-9132-0
de Vries, Hanna & Tsoulos, George. 2023. Portions and countability: A crosslinguistic investigation. Nat Lan Linguistic Theory. DOI: http://doi.org/10.1007/s11049-023-09576-3
Doetjes, Jenny. 1997. Quantifiers and selection: On the distribution of quantifying expressions in French, Dutch and English. Leiden/The Hague: Holland Academic Graphics.
Doetjes, Jenny. 2012. Count/mass distinctions across languages. In Maienborn, Claudia & von Heusinger, Klaus & Portner, Paul (eds.), Semantics: An international handbook of natural language meaning 3. 2559–2580. Berlin: De Gruyter.
Doetjes, Jenny. 2021. Count-mass asymmetries: The importance of being count. In Kiss, Tibor, Pelletier, Francis Jeffry & Husić, Halima (eds.), Things and stuff: The semantics of the count-mass distinction, 81–114. Cambridge: Cambridge University Press. DOI: http://doi.org/10.1017/9781108937979.005
Filip, Hana & Sutton Peter R. 2017. Singular count NPs in measure constructions. In Proceedings of the 27th Conference on Semantics and Linguistic Theory (SALT 27), 340–357. DOI: http://doi.org/10.3765/salt.v27i0.4277
Galucio, Ana Vilacy & Costa, Carla Nascimento. 2020. Count-mass distinction in Sakurabiat. Linguistic Variation 20(2). 336–351. DOI: http://doi.org/10.1075/lv.00025.gal
Gillon, Carrie. 2010. The mass/count distinction in Innu-aimun: Implications for the meaning of plurality. The fifteenth workshop on structure and constituency in Languages of the Americas (WSCLA 15), 12–29.
Greenberg, Joseph. 1974. Language typology: A historical and analytic overview. Berlin: de Gruyter. DOI: http://doi.org/10.1515/9783110886436
Grice, H. Paul. 1975. Logic and conversation. In Cole, Peter & Morgan, Jerry L. (eds.), Syntax and semantics, Vol. 3, Speech Acts, 41–58. New York: Academic Press. DOI: http://doi.org/10.1163/9789004368811_003
Instituto Brasileiro de Geografia e Estatística (IBGE). 2012. Censo demográfico 2010 – Características gerais dos indígenas: resultados do universo. Rio de Janeiro.
Ionin, Tania & Matushansky, Ora. 2006. The composition of complex cardinals. Journal of Semantics 23. 315–360. DOI: http://doi.org/10.1093/jos/ffl006
Krifka, Manfred. 1989. Nominal reference, temporal constitution, and quantification in event semantics. In Bartsch, Renate & van Benthem, Johan & Boas, von Emde (eds.), Semantics and contextual expression, 75–115. DOI: http://doi.org/10.1515/9783110877335-005
Krifka, Manfred. 1995. Common nouns: A contrastive analysis of Chinese and English. In Carlson, Gregory N. & Pelletier, Francis Jeffry (eds.), The generic book, 398–411. Chicago: UCP.
Krifka, Manfred. 2003. Bare NPs: Kind-referring, indefinites, both, or neither? In Young, Robert B. & Zhou, Yuping (eds.), Proceedings of the 13th Conference on Semantics and Linguistic Theory (SALT 13), 180–203. DOI: http://doi.org/10.3765/salt.v13i0.2880
Krifka, Manfred. 2007. Masses and countables: Cognitive and linguistic factors. Talk at CASTL Workshop “The syntax and semantics of measurement”. University of Tromsø, Sept, 17–18.
Krifka, Manfred. 2008. Different kinds of count nouns and plurals. Talk at syntax in the world’s languages III – Freie Universität Berlin – Sept, 25–28.
Krifka, Manfred. 2011. Varieties of semantic evidence. In Maienborn, Claudia & von Heusinger, Klaus & Portner, Paul (eds.), Semantics: An international handbook of natural language meaning, vol. 2. 242–267. Berlin: Mouton de Gruyter.
Landman, Fred. 2020. Iceberg semantics for mass nouns and count nouns: A new framework for Boolean semantics. Studies in linguistics and philosophy, vol. 105. New York: Springer. DOI: http://doi.org/10.1007/978-3-030-42711-5
Lima, Suzi. 2014a. The grammar of individuation and counting. Amherst, MA: University of Massachusetts dissertation.
Lima, Suzi. 2014b. All notional nouns are count nouns in Yudja. Proceedings of the 24th Conference on Semantics and Linguistic Theory (SALT 24), 534–554. DOI: http://doi.org/10.3765/salt.v24i0.2419
Lima, Suzi. 2016. Container constructions in Yudja: Locatives, individuation and measure. The Baltic International YearBook of Cognition, Logic and Communication 11. 1–40. DOI: http://doi.org/10.4148/1944-3676.1109
Lima, Suzi & Rothstein, Susan. 2020. A typology of the mass/count distinction in Brazil and its relevance for mass/count theories. Linguistic Variation 20(2). 174–218. DOI: http://doi.org/10.1075/lv.20.2
Marantz, Alec. 1997. No escape from syntax: Don’t try morphological analysis in the privacy of your own lexicon. Proceedings of the 21st Penn Linguistics Colloquium, UPenn Working Papers in Linguistics, 201–225.
Marantz, Alec. 2001. Words and things. handout, MIT.
Mathieu, Eric. 2012. On the mass/count distinction in Ojibwe. In Massam, Diane (ed.), Count and mass across languages, 172–98. Oxford: Oxford University Press. DOI: http://doi.org/10.1093/acprof:oso/9780199654277.003.0010
Matthewson, Lisa. 2004. On the methodology of semantic fieldwork. International Journal of American linguistics 70(4). 369–415. DOI: http://doi.org/10.1086/429207
Matthewson, Lisa. 2012. A fieldworker’s guide to the semantics of noun phrases. Talk given at Amazonicas 4, Pontificia Universidad Católica del Peru, Lima.
Moravcsik, Edith A. 2017. Number. In Aikhenvald, Alexandra Y. & Dixon, Robert M.W. (eds.), The Cambridge handbook of linguistic typology, 440–476. Cambridge University Press. DOI: http://doi.org/10.1017/9781316135716.014
Nascimento, Silvia Helena Lovato do. 1995. Aspectos morfológicos e sintáticos da marcação de caso da língua Kaingang. Santa Catarina, SC: The State University of Santa Catarina dissertation.
Pelletier, Francis Jeffry. 1975. Non-singular reference: Some preliminaries. Philosophia 5(4). 451–465. DOI: http://doi.org/10.1007/BF02379268
Pires de Oliveira, Roberta & Rothstein, Susan. 2011. Bare singular noun phrases are mass in Brazilian Portuguese. Lingua 121(15). 2153–2175. DOI: http://doi.org/10.1016/j.lingua.2011.09.004
Quine, Willard Van Orman. 1960. Word and object. Cambridge: MIT Press.
Reinhart, Tanya. 1997. Quantifier scope: How labor is divided between QR and choice functions. Linguistics and Philosophy 20(4). 335–397. DOI: http://doi.org/10.1023/A:1005349801431
Rothstein, Susan. 2007. Counting and the mass-count distinction. Ms. Bar-Ilan University.
Rothstein, Susan. 2010. Counting and the mass/count distinction. Journal of Semantics 24. 343–397. DOI: http://doi.org/10.1093/jos/ffq007
Rothstein, Susan. 2016. Object mass nouns from a crosslinguistic perspective. Talk given at Workshop: Syntax and Semantics of the Nominal Domain. Goethe-University, Frankfurt, February 4.
Rothstein, Susan. 2017. Semantics for counting and measuring. Cambridge: Cambridge University Press. DOI: http://doi.org/10.1017/9780511734830
Rothstein, Susan. 2021. Counting, plurality, and portions. In Kiss, Tibor, Pelletier, Francis Jeffry & Husić, Halima (eds.), Things and stuffs: The semantics of count-mass distinction, 55–79. Cambridge: Cambridge University Press. DOI: http://doi.org/10.1017/9781108937979.004
Rothstein, Susan & Pires de Oliveira, Roberta. 2016. Comparatives in Brazilian Portuguese: Counting and measuring. In Moltmann, Friederike (ed.), Mass and count in linguistics, philosophy, and cognitive science, 141–158. John Benjamins: Amsterdam. DOI: http://doi.org/10.1075/lfab.16.07rot
Sanchez-Mendes, Luciana & Gomes, Ana Paula Quadros & Aronaldo, Julio. 2020. The count-mass distinction in Terena. Linguistic Variation 20(2). 382–397. DOI: http://doi.org/10.1075/lv.00028.san
Schwarzschild. Roger. 1996. Pluralities. Studies in Linguistics and Philosophy 61. Kluwer Academic Publishers. DOI: http://doi.org/10.1007/978-94-017-2704-4
Schwarzschild, Roger. 2011. Stubborn distributivity, multiparticipant nouns and the count/mass distinction. Proceedings of the 39th Meeting of the Northeast Linguistic Society (NELS 39) 2. 661–678.
Wiesemann, Ursula. 1971. Kaingáng-Português, Português-Kaingáng. Brasília: Summer Institute of Linguistics/Funai.
Wiesemann, Ursula. 2002. Kaingang-Português: Dicionário bilíngue. Curitiba: Editora Evangélica Esperança.
Wilhelm, Andrea. 2008. Bare nouns and number in Dëne Sųłiné. Natural Language Semantics 16. 39–68. https://www.jstor.org/stable/23749498. DOI: http://doi.org/10.1007/s11050-007-9024-9.
Wiltschko, Martina. 2012. Decomposing the mass/count distinction: Evidence from languages that lack it. In Massam, Diane (ed.), Count and mass across languages, 120–46. Oxford: Oxford University Press. DOI: http://doi.org/10.1093/acprof:oso/9780199654277.003.0009
Winter, Yoad. 1997. Choice Functions and the Scopal Semantics of Indefinites. Linguistics and Philosophy 20(4). 399–467. https://www.jstor.org/stable/25001672. DOI: http://doi.org/10.1023/A:1005354323136